MGEM
3D Modelling
OF
Highly Conductive Massive Sulphide Bodies
Owen Fernley
MGEM
3D Forward Modelling Tool
- Large loop and large volume EM problems
- High contrast resistivity
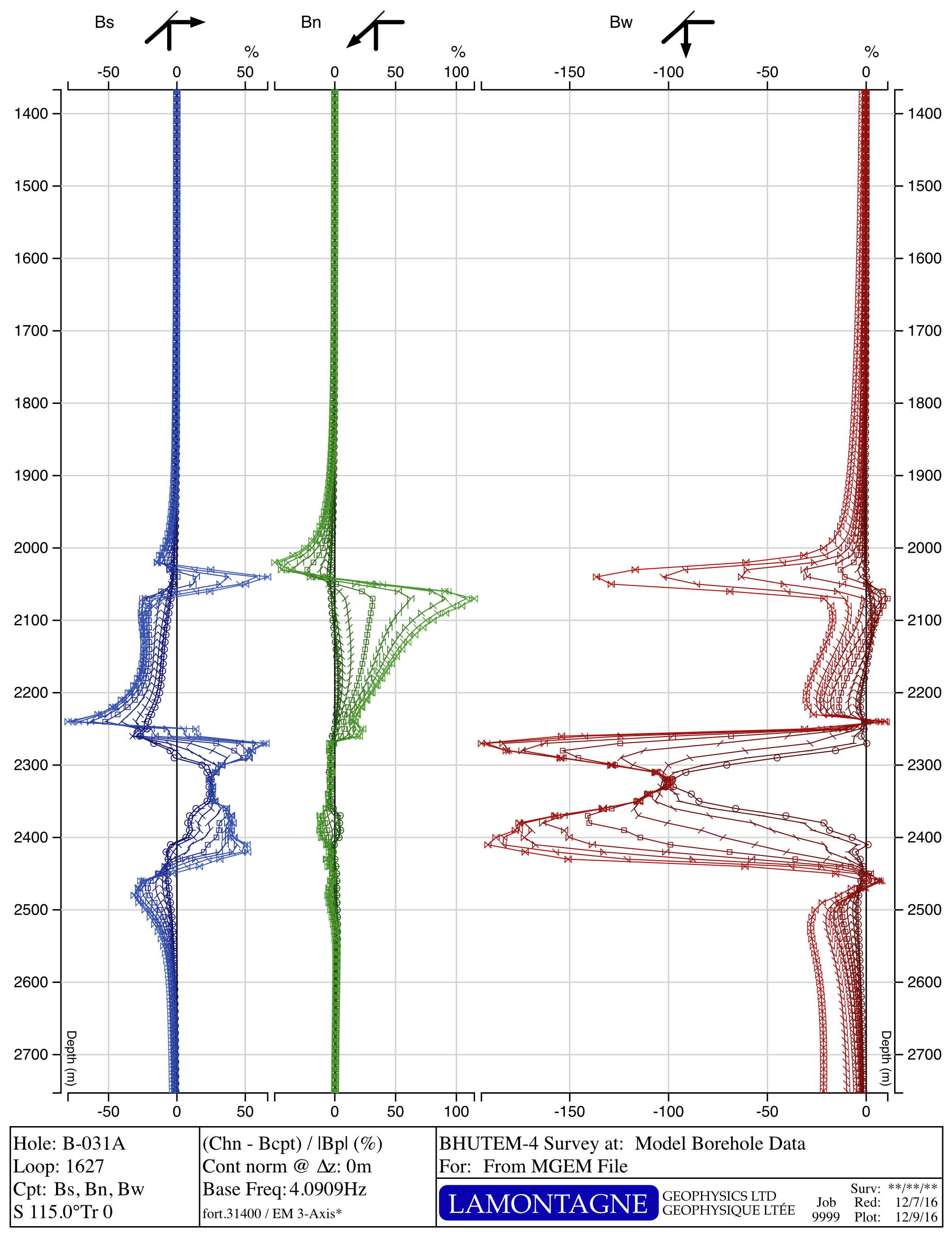
- Surface and downhole survey modelling
- Ideal for massive sulphide exploration
MGEM
Finite Difference 3D EM Modelling
- Finite Difference Equation
- The Staggered Grid
- Boundary Conditions
- Multigrid Solver
MGEM
Results and Case Studies
- Synthetic Sphere
- Voisey's Bay Ovoid
- Mystery Project
Part 1
Finite Difference 3D EM Modelling
Equation to Solve
$ (\vec{\nabla} \times \color{orange}{\rho} \vec{\nabla} \times \color{fuchsia}{\vec{\mathrm{H}}}) + \frac{\partial\mu_0\color{fuchsia}{\vec{\mathrm{H}}}}{\partial t} = -\frac{\partial\vec{\mathrm{B}}_p}{\partial t} $
Time Stepping
$ \vec{\mathrm{H}}_i = \vec{\mathrm{H}}_{i-1} + \vec{\mathrm{D}} $
Maxwell's Equations
$ \vec{\nabla} \bullet \vec{\mathrm{E}} = \frac{\rho}{\varepsilon_0} $
$ \vec{\nabla} \bullet \vec{\mathrm{B}} = 0 $
$ \vec{\nabla} \times \vec{\mathrm{E}} = -\frac{\partial\vec{\mathrm{B}}}{\partial t} $
$ \vec{\nabla} \times \vec{\mathrm{B}} = \mu_0 \left(\vec{\mathrm{J}} + \varepsilon_0\frac{\partial\vec{\mathrm{E}}}{\partial t}\right) $
Current Density
$ \color{red}{\vec{\mathrm{E}}} = \color{orange}{\rho}\vec{\mathrm{J}} $
Faraday's Law
$ \vec{\nabla} \times \color{red}{\vec{\mathrm{E}}} = -\frac{\partial\vec{\mathrm{B}}}{\partial t} $
Ampere's Law
$ \vec{\nabla} \times \vec{\mathrm{B}} = \mu_0 \vec{\mathrm{J}} $
Current Density
$ \color{grey}{\vec{\mathrm{E}} = \rho\vec{\mathrm{J}}} $
Faraday's Law
$ \vec{\nabla} \times \color{orange}{\rho}\vec{\mathrm{J}} = -\frac{\partial\vec{\mathrm{B}}}{\partial t} $
Ampere's Law
$ \vec{\nabla} \times \vec{\mathrm{B}} = \mu_0 \vec{\mathrm{J}} $
Current Density
$ \color{grey}{\vec{\mathrm{E}} = \rho\vec{\mathrm{J}}} $
Faraday's Law
$ \vec{\nabla} \times \color{orange}{\rho}\vec{\mathrm{J}} = -\frac{\partial\vec{\mathrm{B}}}{\partial t} $
Ampere's Law
$ \vec{\nabla} \times \color{fuchsia}{\vec{\mathrm{B}}} = \color{fuchsia}{\mu_0} \vec{\mathrm{J}} $
Current Density
$ \color{grey}{\vec{\mathrm{E}} = \rho\vec{\mathrm{J}}} $
Faraday's Law
$ \vec{\nabla} \times \color{orange}{\rho}\color{green}{\vec{\mathrm{J}}} = -\frac{\partial\vec{\mathrm{B}}}{\partial t} $
Ampere's Law
$ \vec{\nabla} \times \color{fuchsia}{\vec{\mathrm{H}}} = \color{green}{\vec{\mathrm{J}}} $
Current Density
$ \color{grey}{\vec{\mathrm{E}} = \rho\vec{\mathrm{J}}} $
Faraday's Law
$ \vec{\nabla} \times \color{orange}{\rho} \vec{\nabla} \times \color{fuchsia}{\vec{\mathrm{H}}} = -\frac{\partial\vec{\mathrm{B}}}{\partial t} $
Ampere's Law
$ \color{grey}{\vec{\nabla} \times \vec{\mathrm{H}} = \vec{\mathrm{J}}} $
Ampere's Law $\longrightarrow$ Faraday's Law
$ \vec{\nabla} \times \color{orange}{\rho} (\vec{\nabla} \times \color{fuchsia}{\vec{\mathrm{H}}}) = -\frac{\partial\vec{\mathrm{B}}}{\partial t} $
Secondary Primary Field
$ \vec{\nabla} \times \color{orange}{\rho} (\vec{\nabla} \times \color{fuchsia}{\vec{\mathrm{H}}}) = \left( \frac{\partial\mu_0\color{fuchsia}{\vec{\mathrm{H}}}}{\partial t} - \frac{\partial\vec{\mathrm{B}}_p}{\partial t} \right) $
Equation to Solve
$ (\vec{\nabla} \times \color{orange}{\rho} \vec{\nabla} \times \color{fuchsia}{\vec{\mathrm{H}}}) + \frac{\partial\mu_0\color{fuchsia}{\vec{\mathrm{H}}}}{\partial t} = -\frac{\partial\vec{\mathrm{B}}_p}{\partial t} $
Time Stepping
$ \color{fuchsia}{\vec{\mathrm{H}}}_i = \color{fuchsia}{\vec{\mathrm{H}}}_{i-1} + \color{fuchsia}{\vec{\mathrm{D}}} $
Finite Difference Equaton
$ \vec{\nabla} \times \color{orange}{\rho} \vec{\nabla} \times \vec{\alpha}\color{fuchsia}{\vec{\mathrm{D}}} + \frac{\mu_0\color{fuchsia}{\vec{\mathrm{D}}}}{h_t} = -\frac{\partial\vec{\mathrm{B}}_p}{\partial t} - \vec{\nabla} \times \color{orange}{\rho}\vec{\nabla} \times\color{fuchsia}{\vec{\mathrm{H}}} $
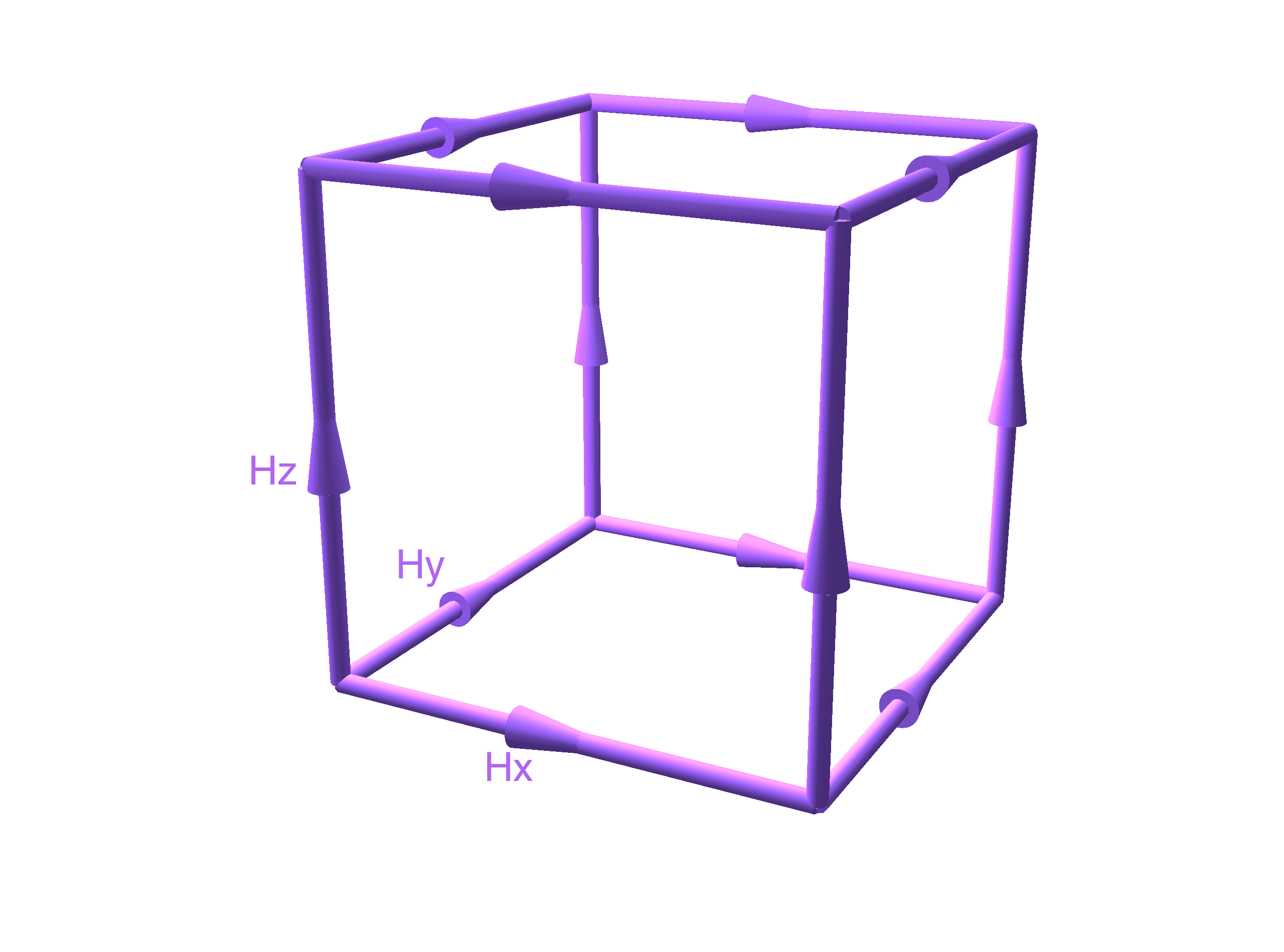
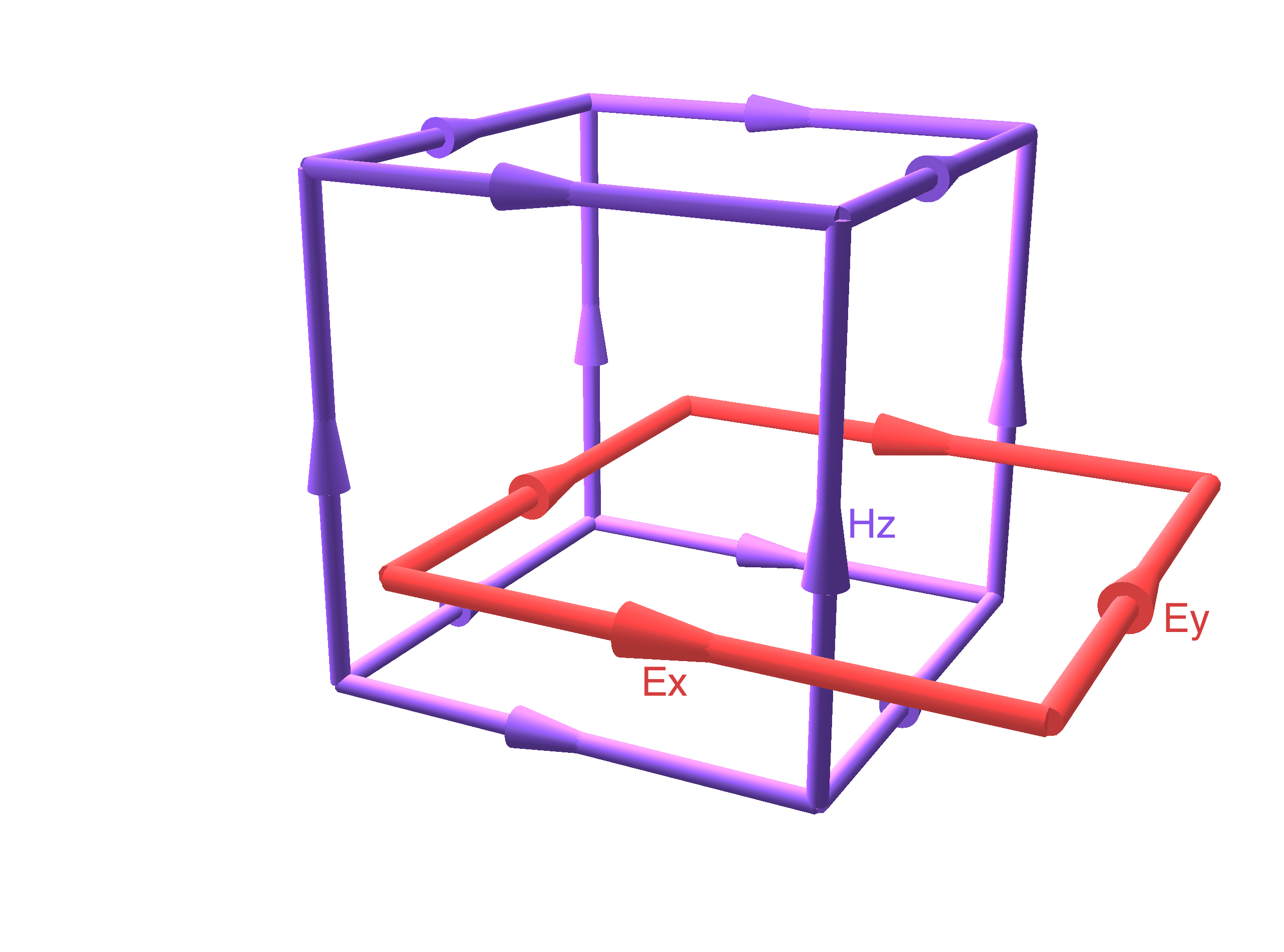
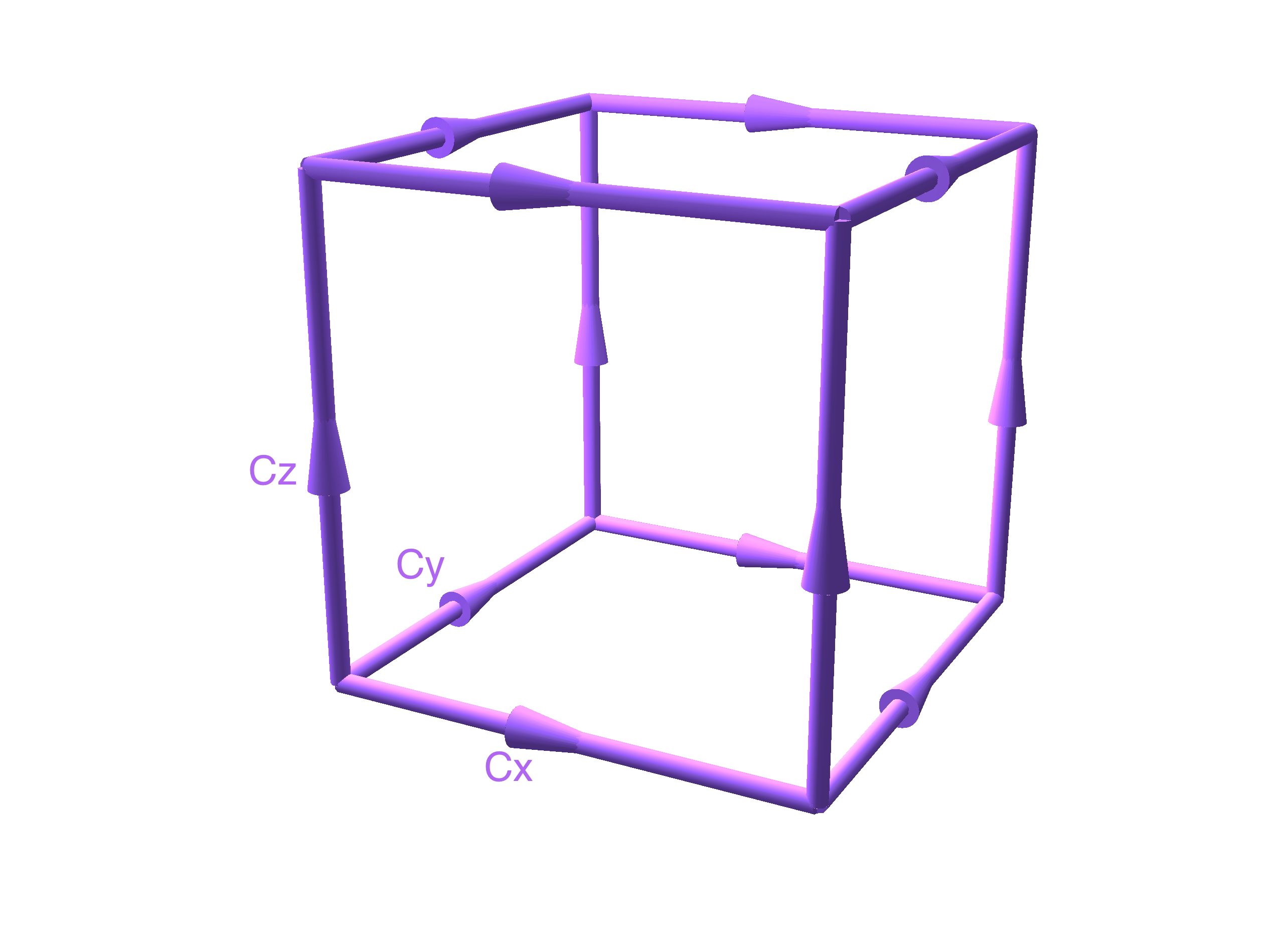
The Staggered Grid
H-Field
E-Field
Conductivity Subgrid
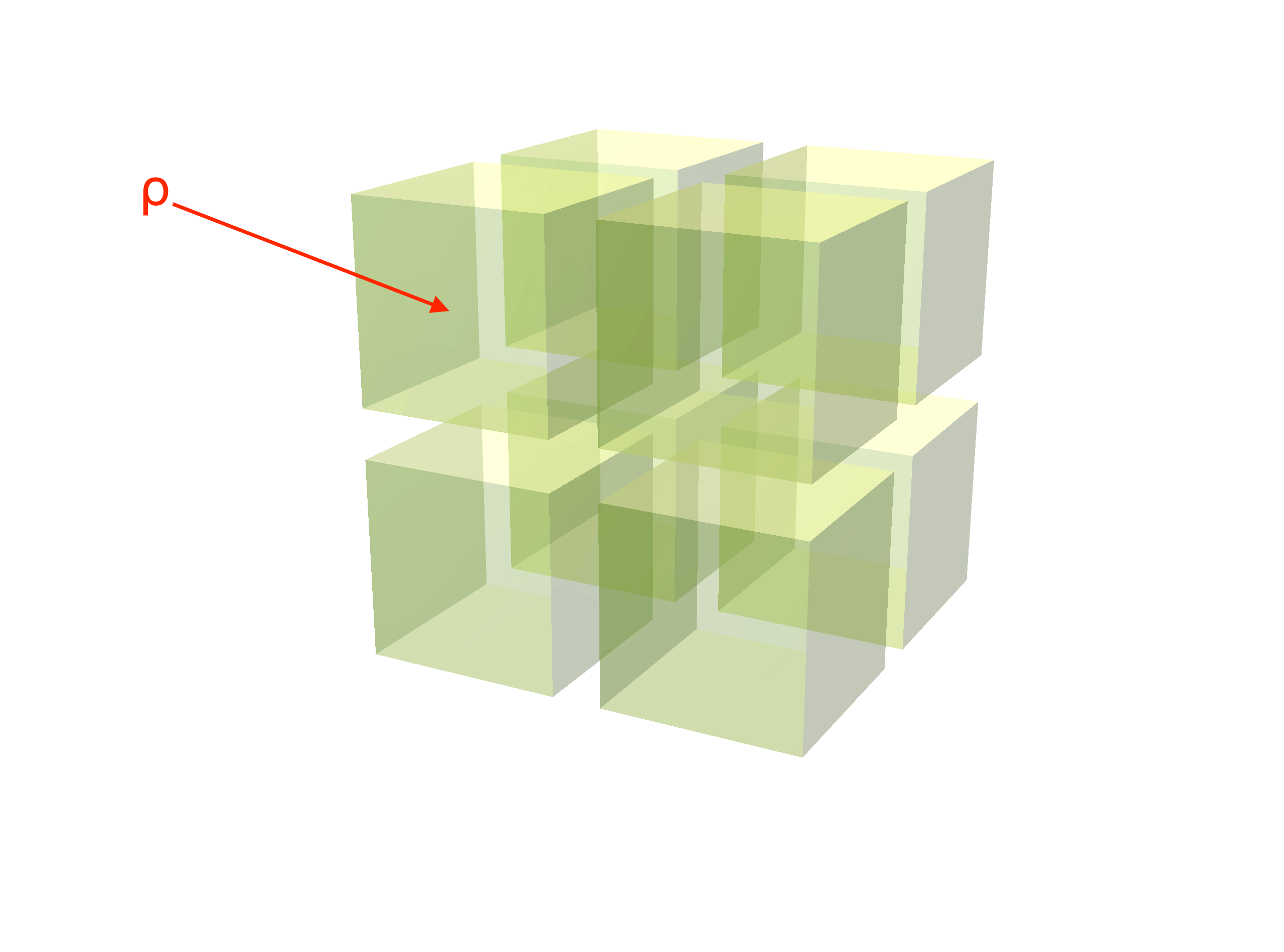
Conductivity Subgrid

Current Density
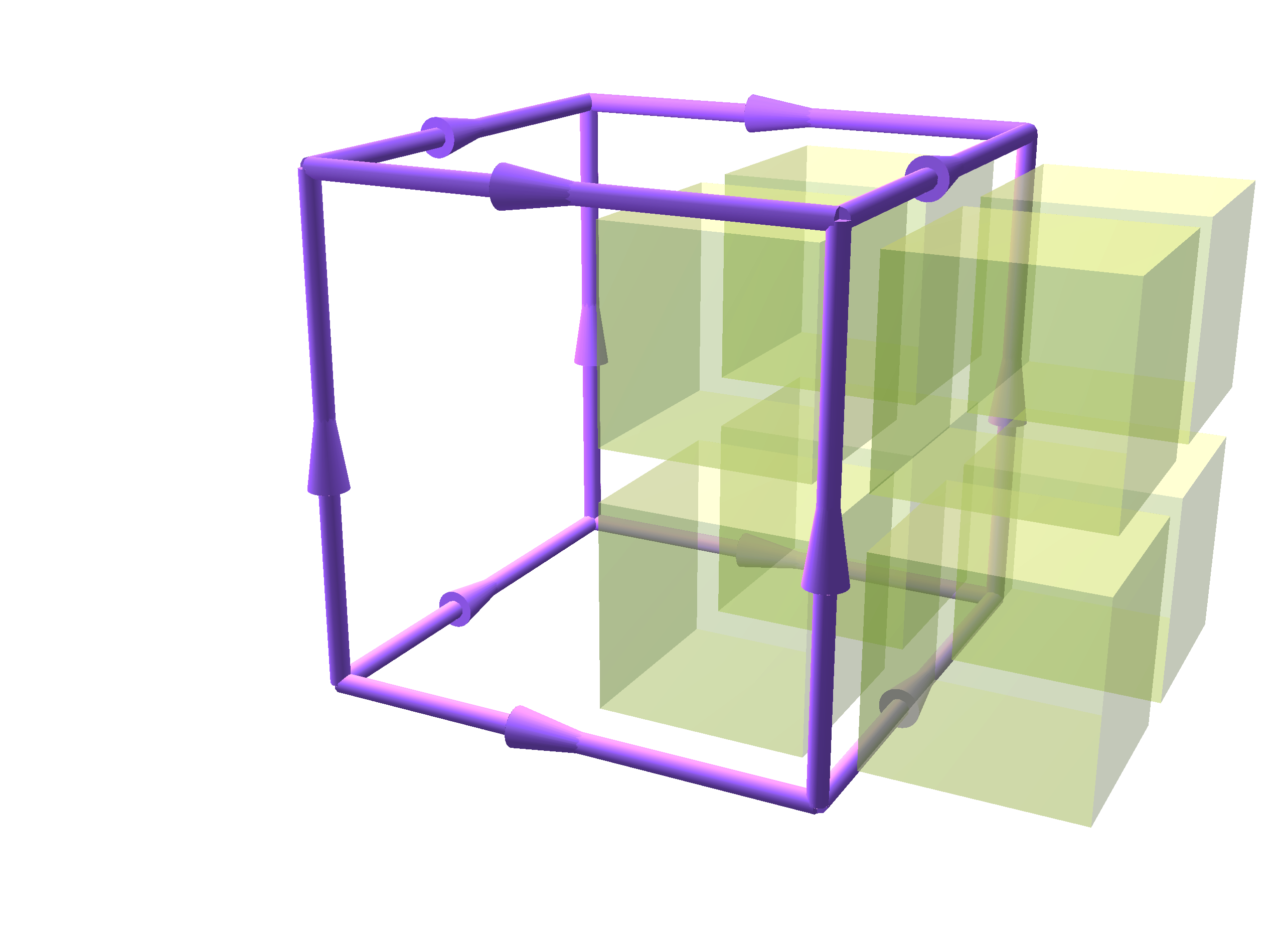
Current Density
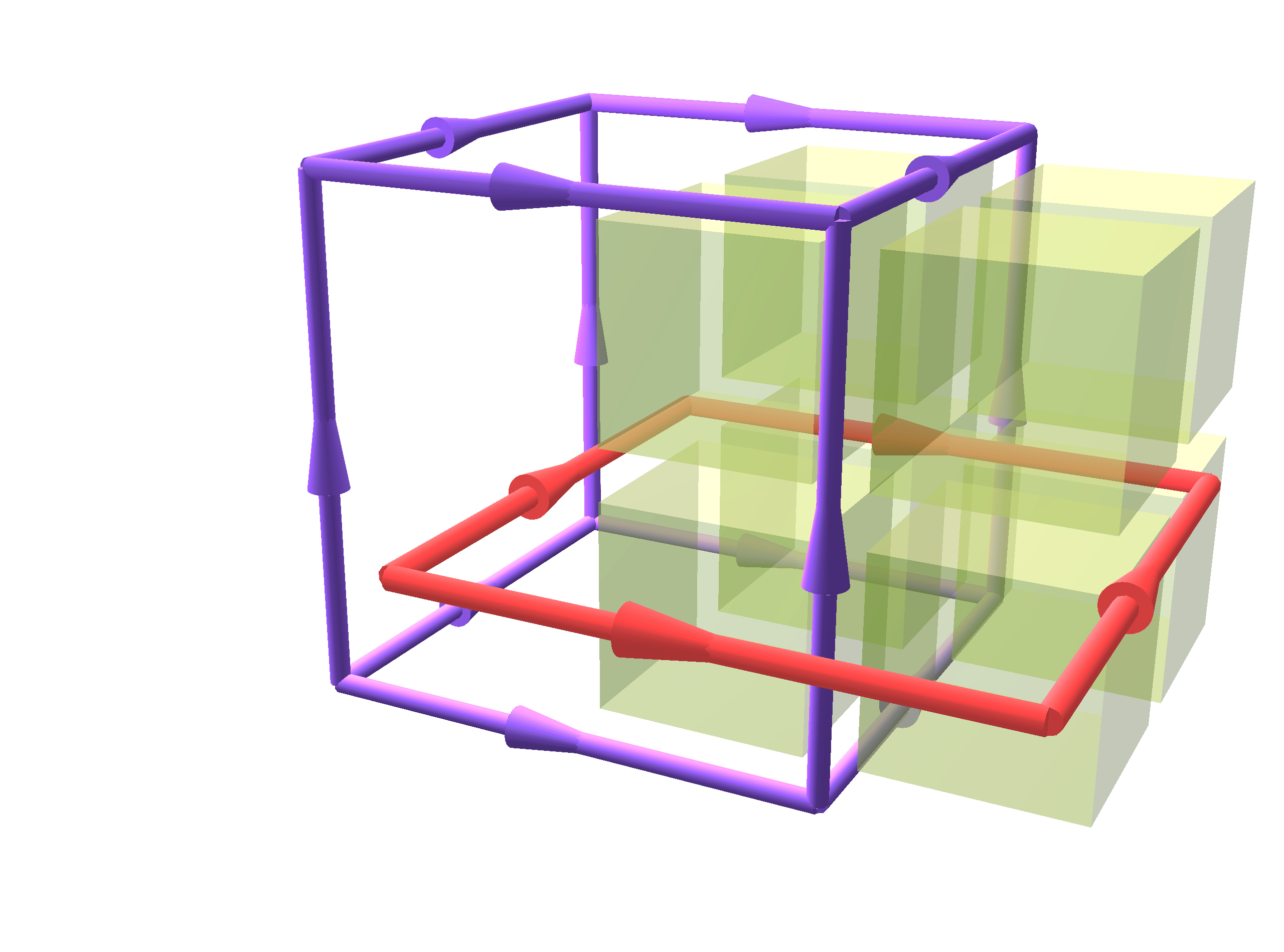
The Staggered Grid
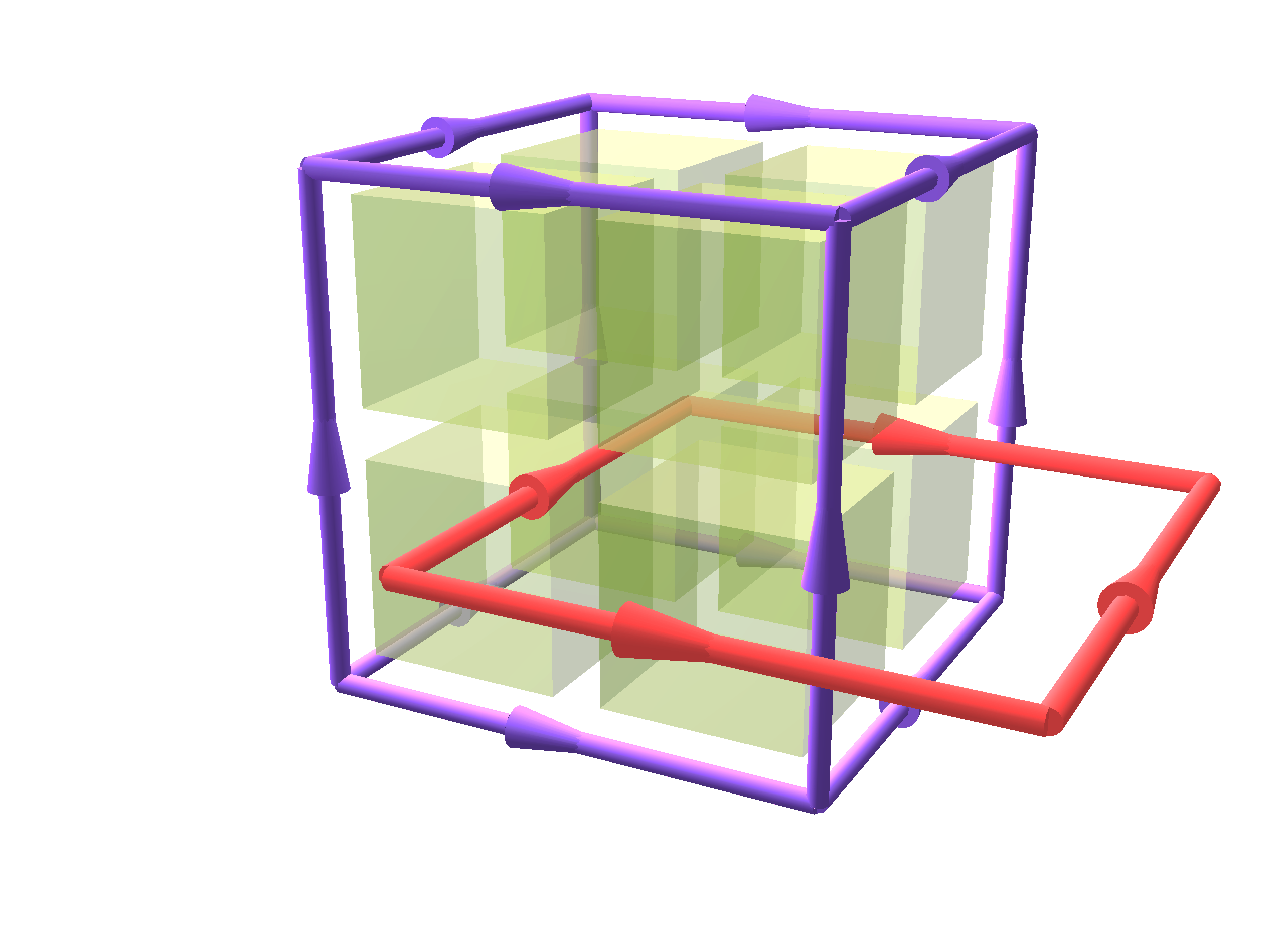
Boundary Conditions
Suitable Initial and Boundary Condition
- $\vec{\mathrm{C}}$ and $\vec{\mathrm{J}}$ start at zero
- $\vec{\mathrm{C}}$ steps on at $t=0$
- $\vec{\mathrm{C}}$ and $\vec{\mathrm{J}}$ decay to zero at infinity
Zero Divergence: The $ \vec{\mathrm{C}} $ Vector
$ \vec{\mathrm{H}} = \vec{\mathrm{C}} - \vec{\nabla}\mathrm{g} $
$ \vec{\mathrm{H}} \longrightarrow \vec{\mathrm{C}} $ provided that $ \vec{\nabla} \bullet \vec{\mathrm{H}} = 0 $
Below Surface
H-Field

C-Field Below Surface
in Free air
$ \vec{\mathrm{H}} = \vec{\nabla}\mathrm{g} $
at surface
$\vec{\mathrm{H}} = \vec{\mathrm{C}} - \vec{\nabla}\mathrm{g} $
below surface
$\vec{\mathrm{H}} = \vec{\mathrm{C}}$
Outer Edge
$\vec{\mathrm{H}_t} = 0$
Outer boundary can be pushed kilometers away
Multigrid Solver
- Speeds up iteration time
- Boundary conditions pushed far from area of interest
- V and W cycle iterations: Interpolation and Sampling
Part 2
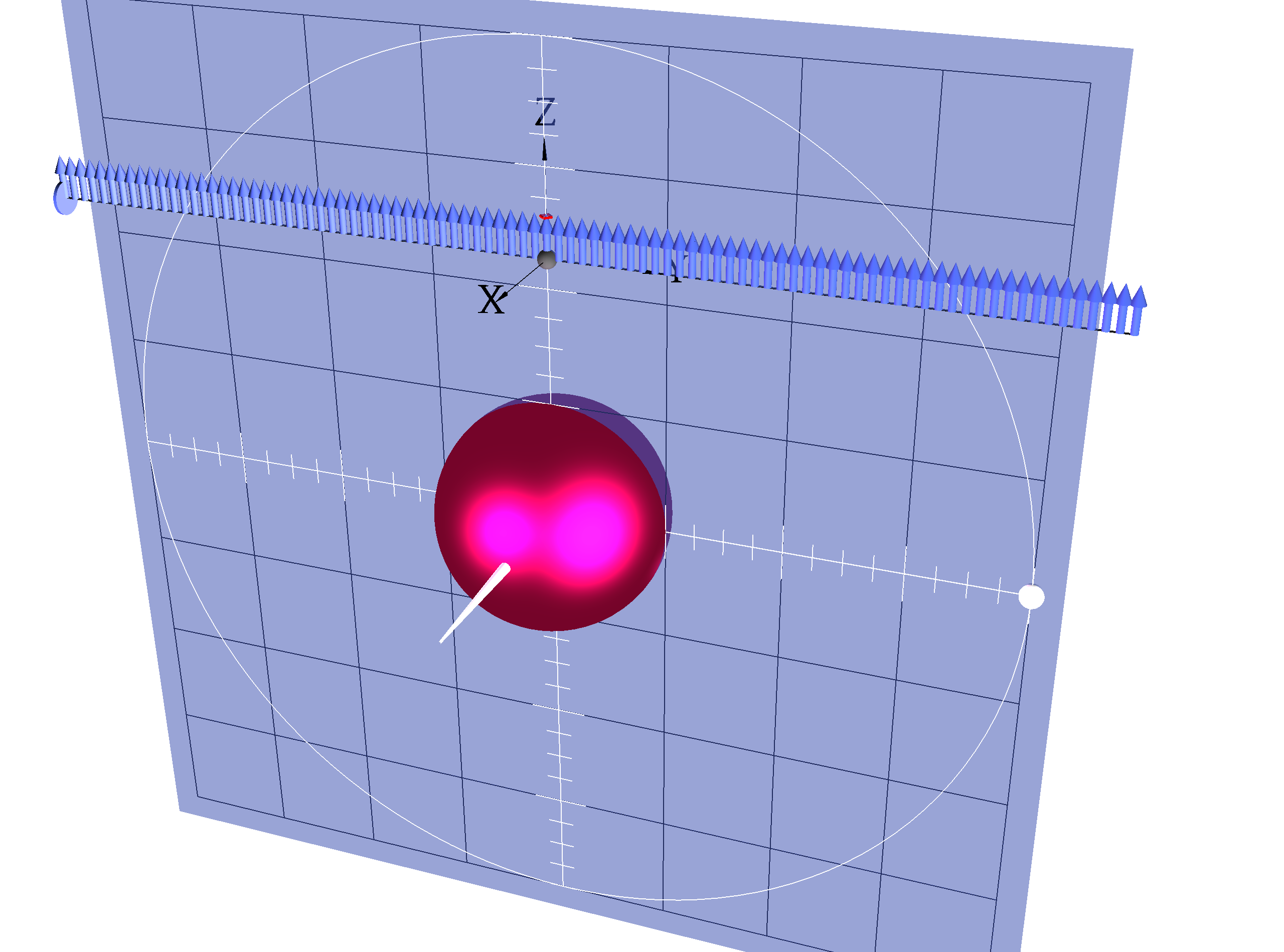
Results and Case Studies
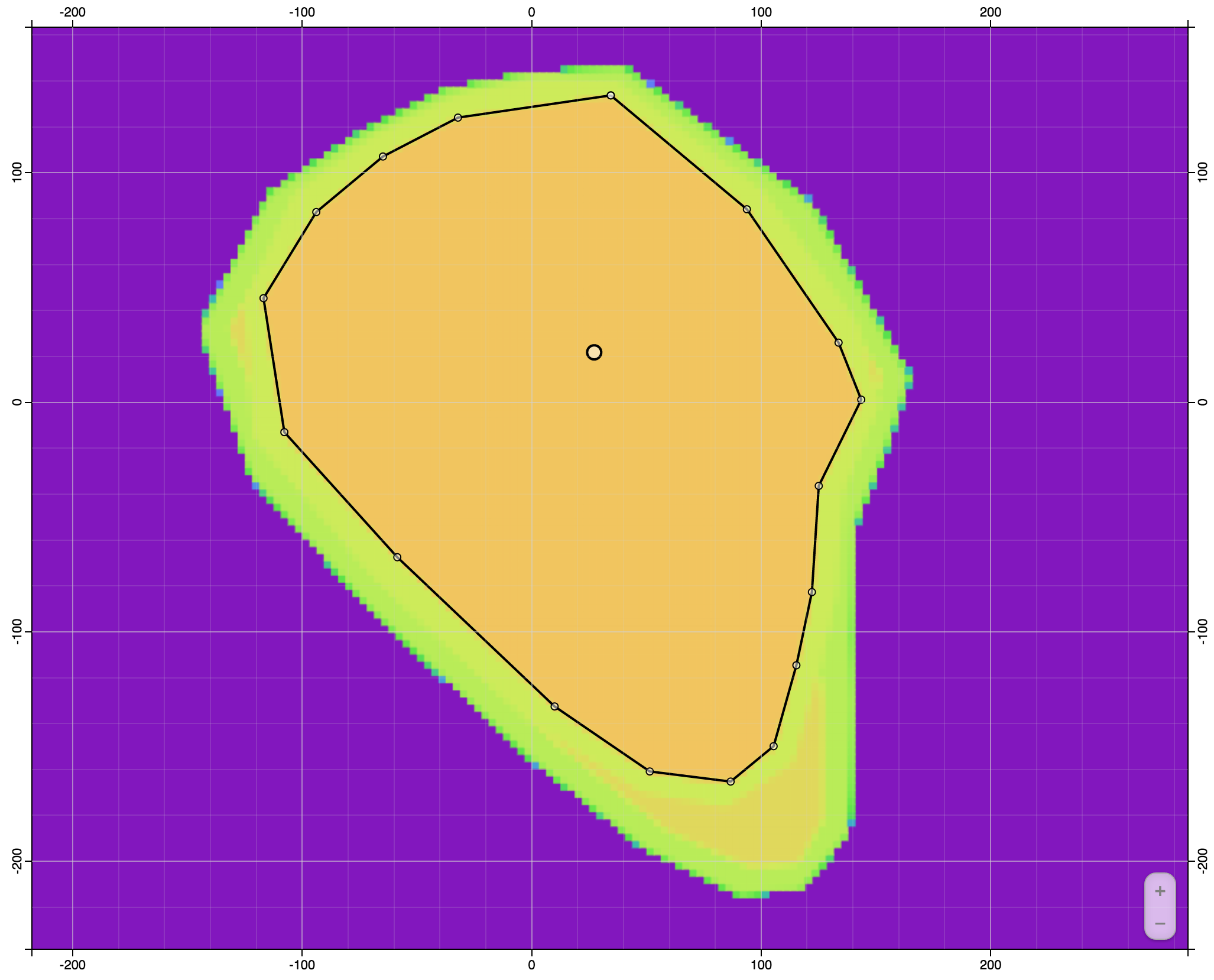
Sphere Test (r = 100 m)

MGEM Software Components
- Gridplot
- 3D Viewer
- MGEM Engine
Model Specifications
follow the law of geologic superposition
Grid Plot
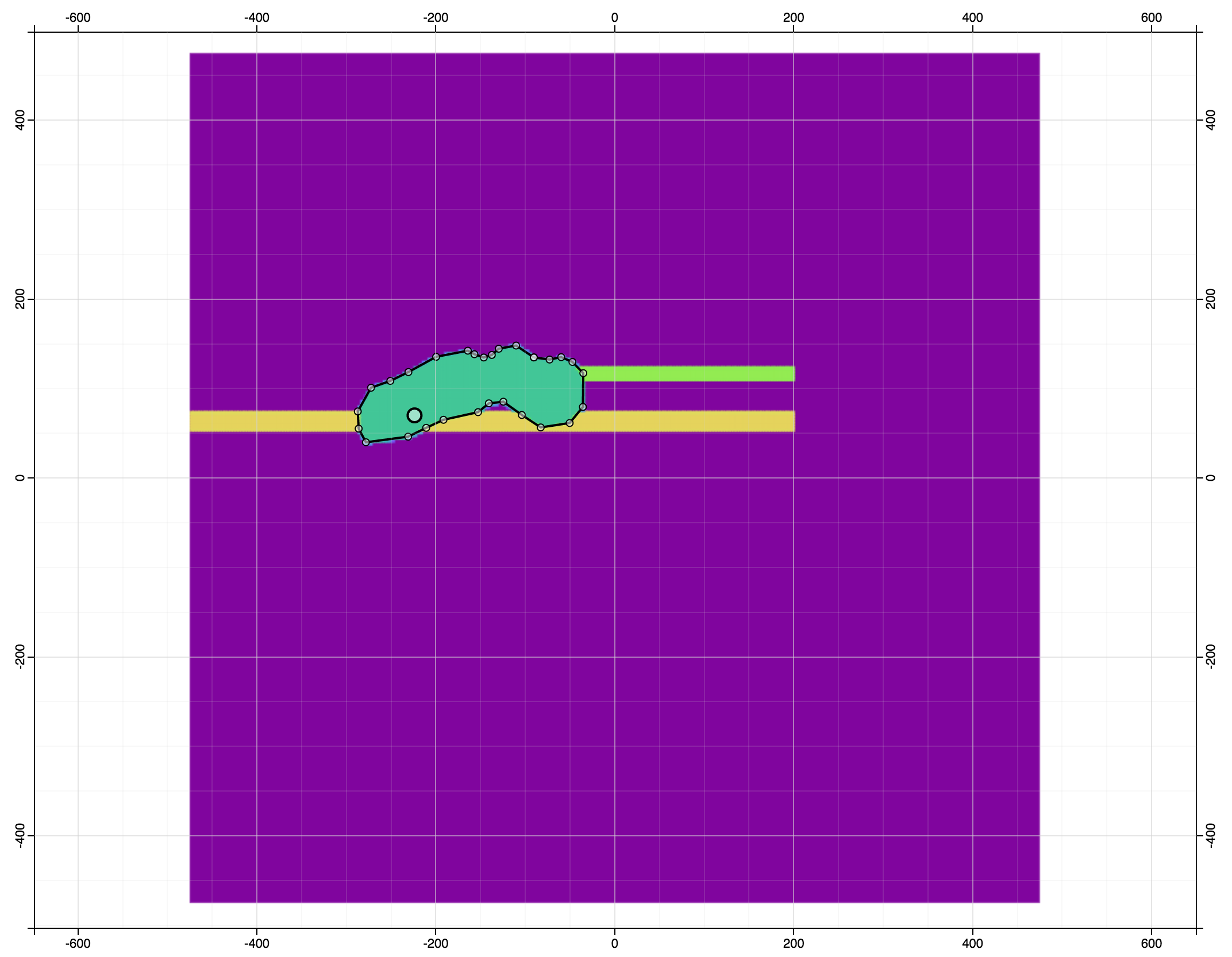
superposition
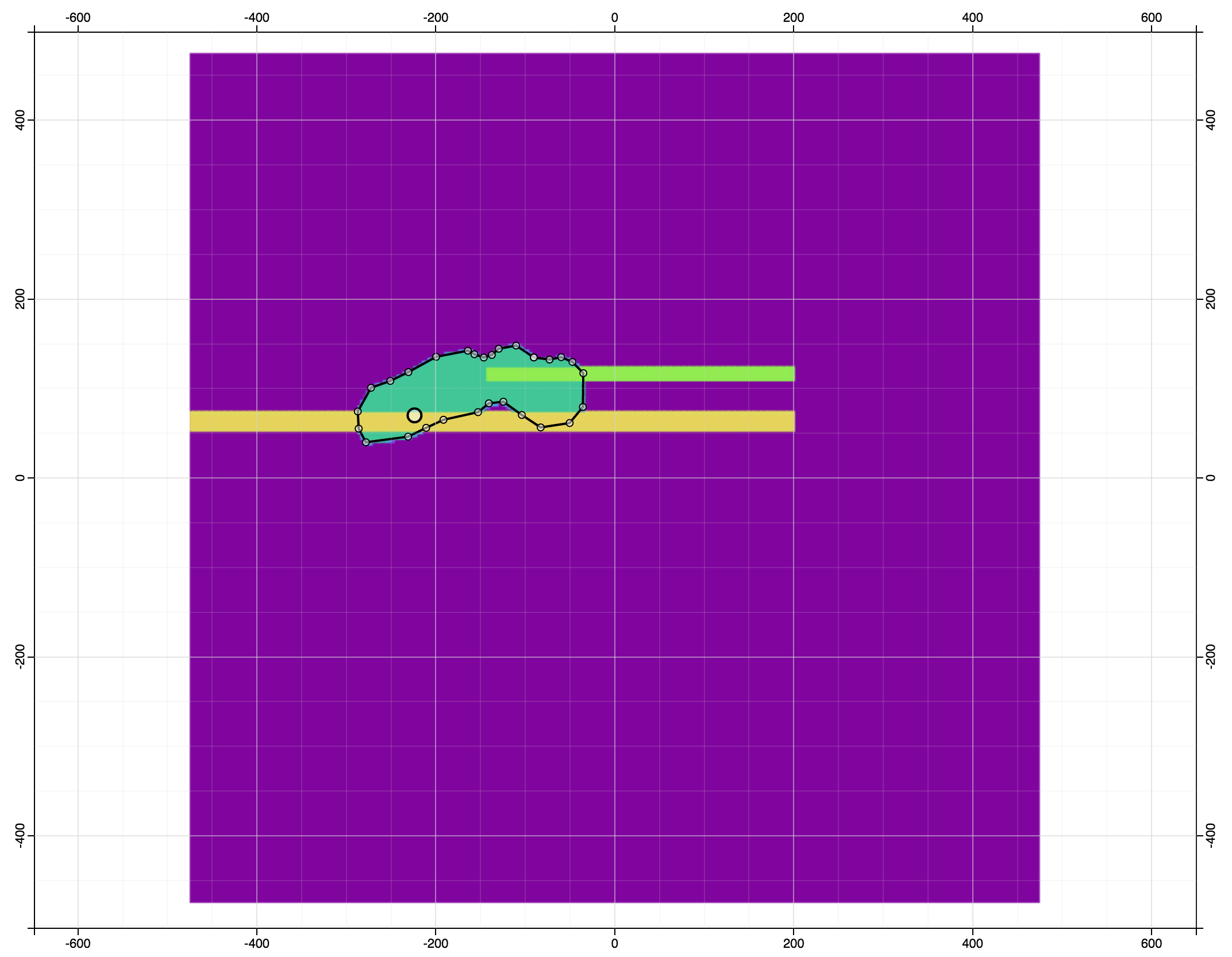
paths

3D Viewer
MGEM Engine
Four runs at once
Composing Models
- background resistivity
- cuboids
- ellipsoids
- polygonal prisms
Cuboids
- dipping layered earths
- quarter space
- overburden
- conductive or resistive dykes
Ellipsoids
ideal for lenses
Polygonal Prisms
detailing known bodies
All together now
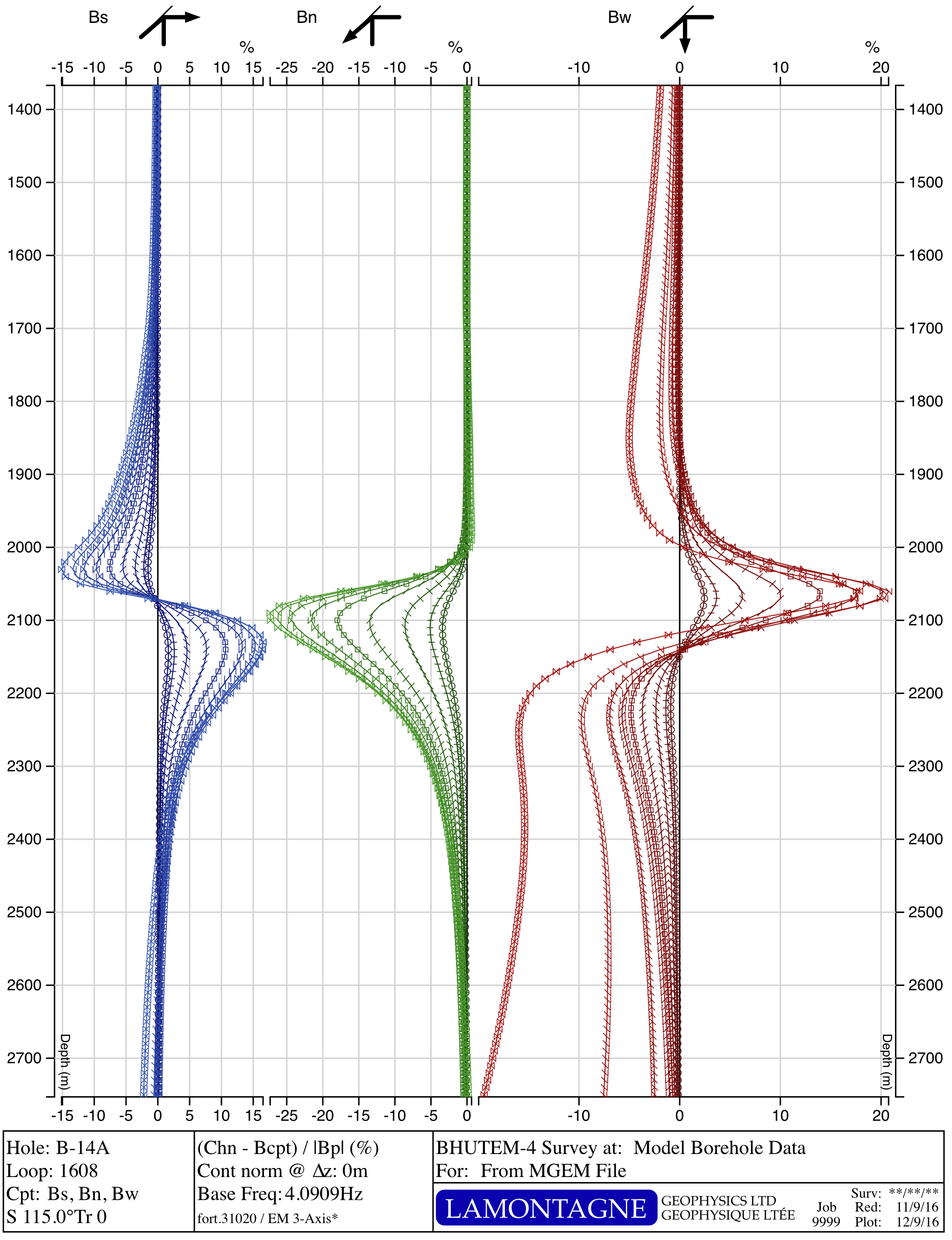
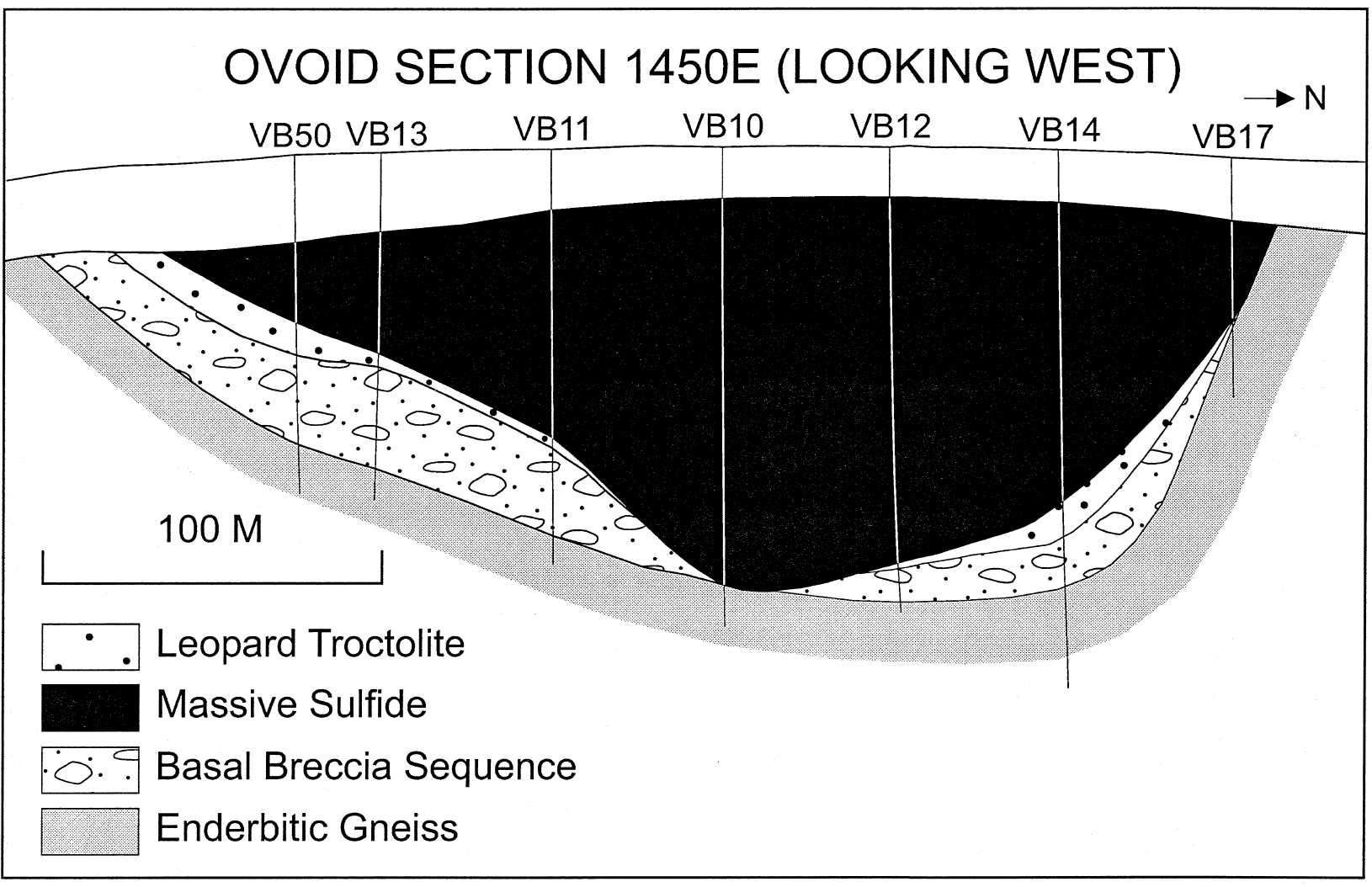
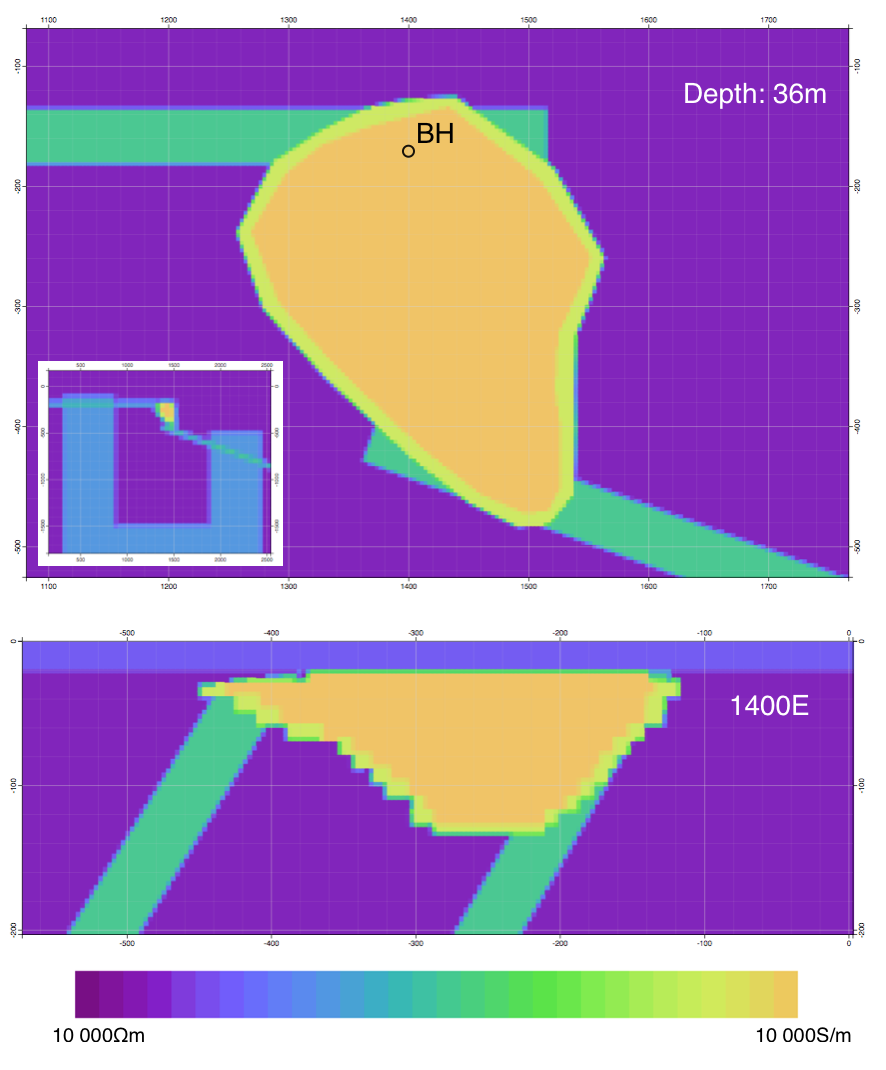
Voisey's Bay Ovoid


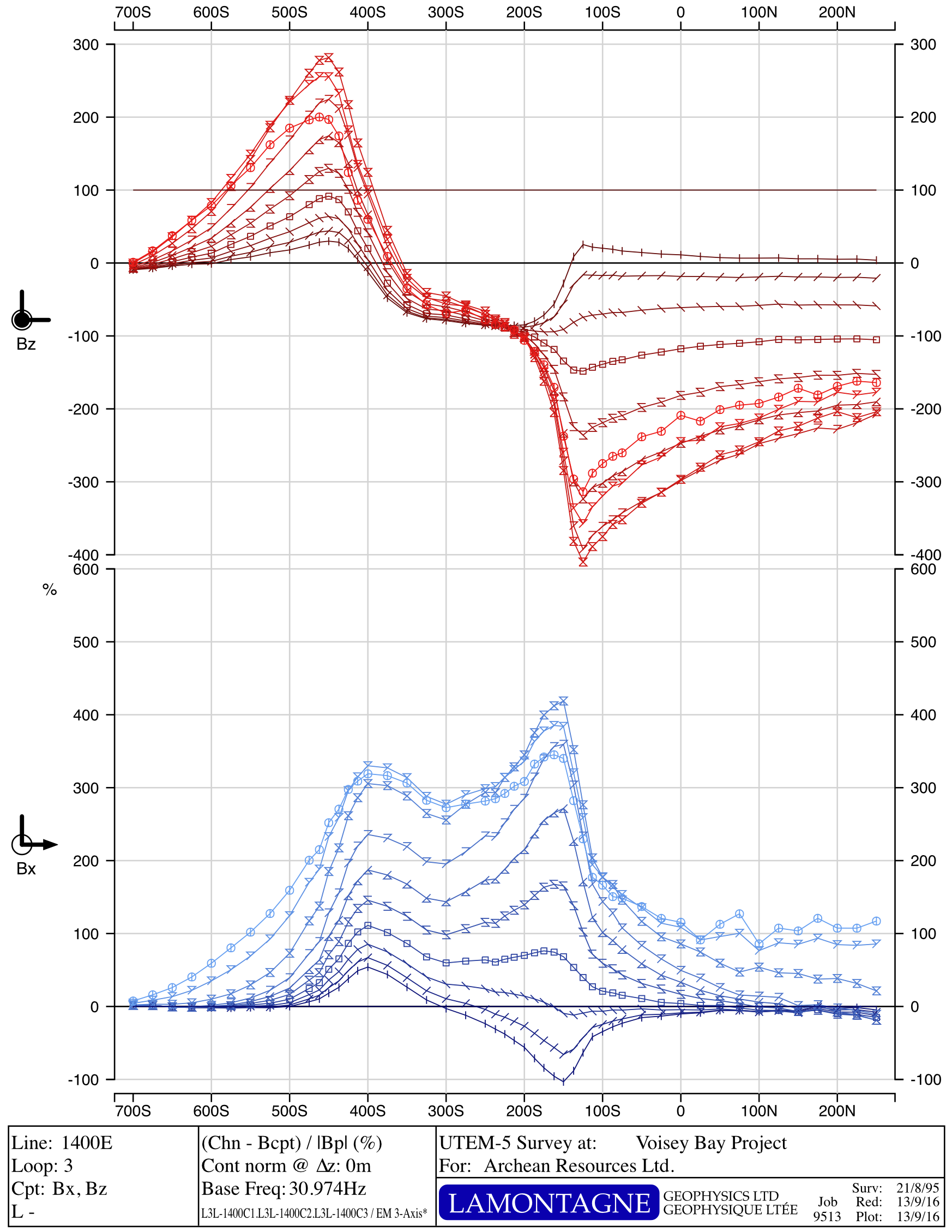
Iterative Forward Modelling
- Ovoid Only
- Overburden
- Troctolyte Dykes
- Conductive Conduit
Ovoid Image Source
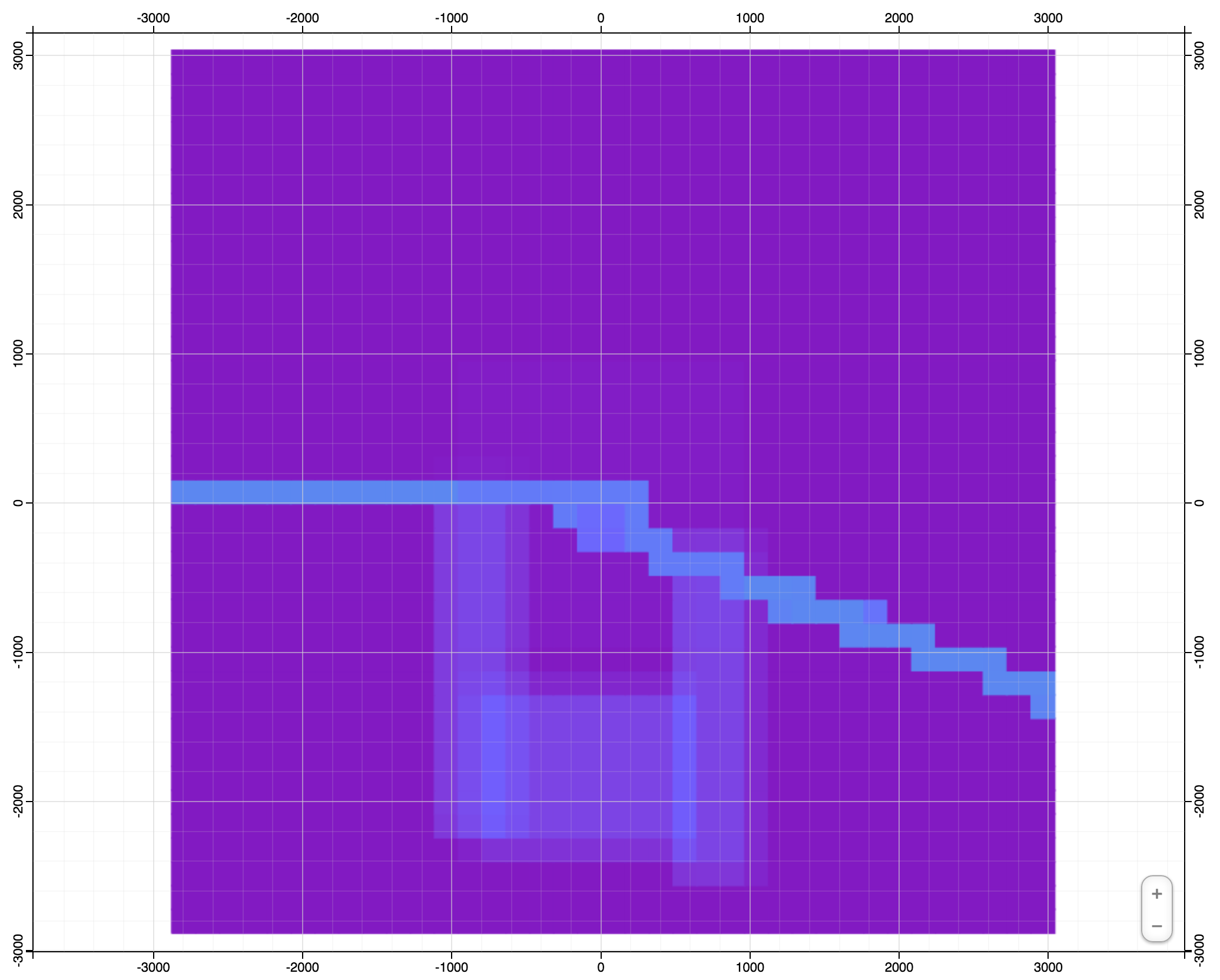
Gridplot Overlay

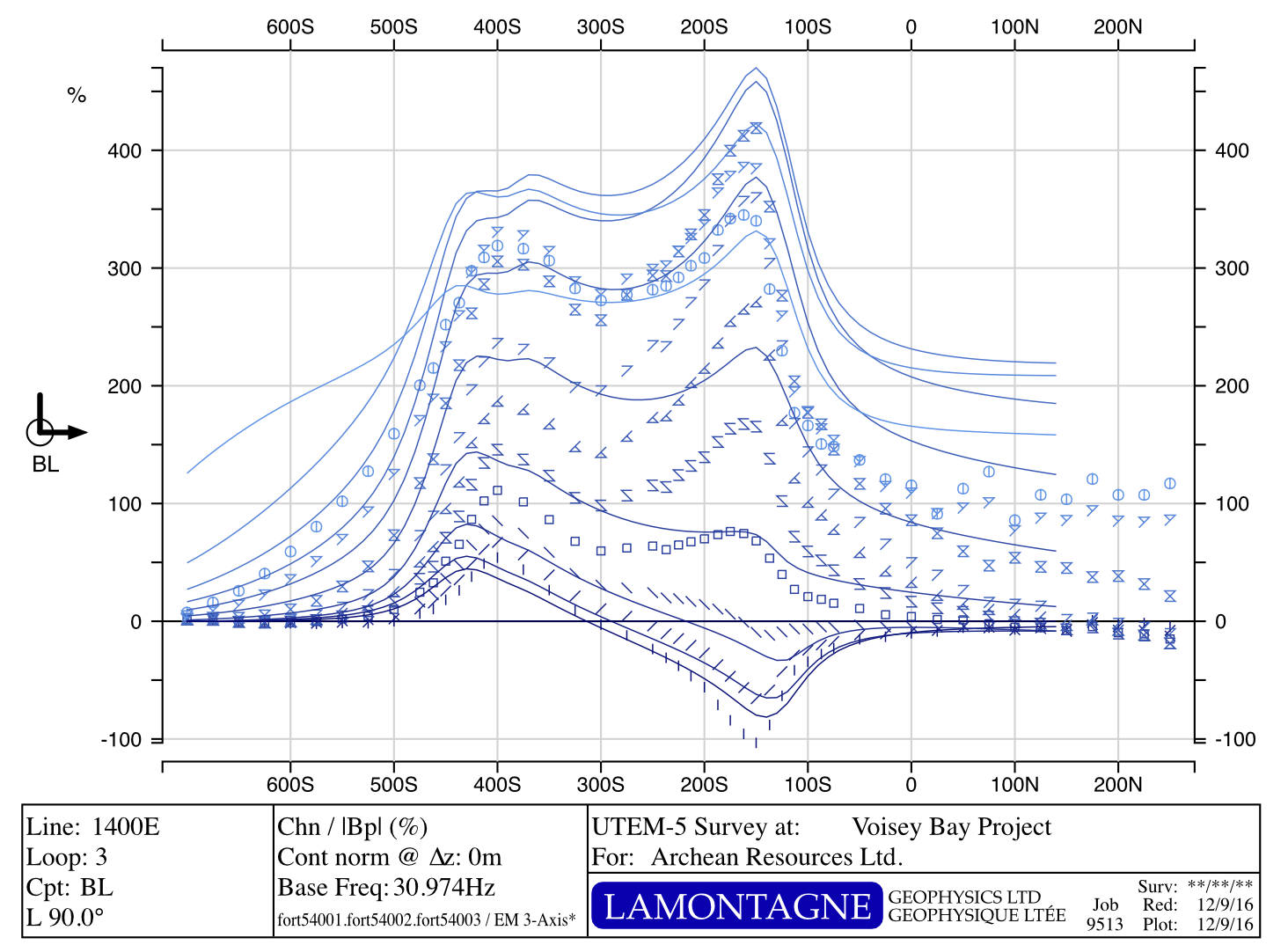
Ovoid X-Section
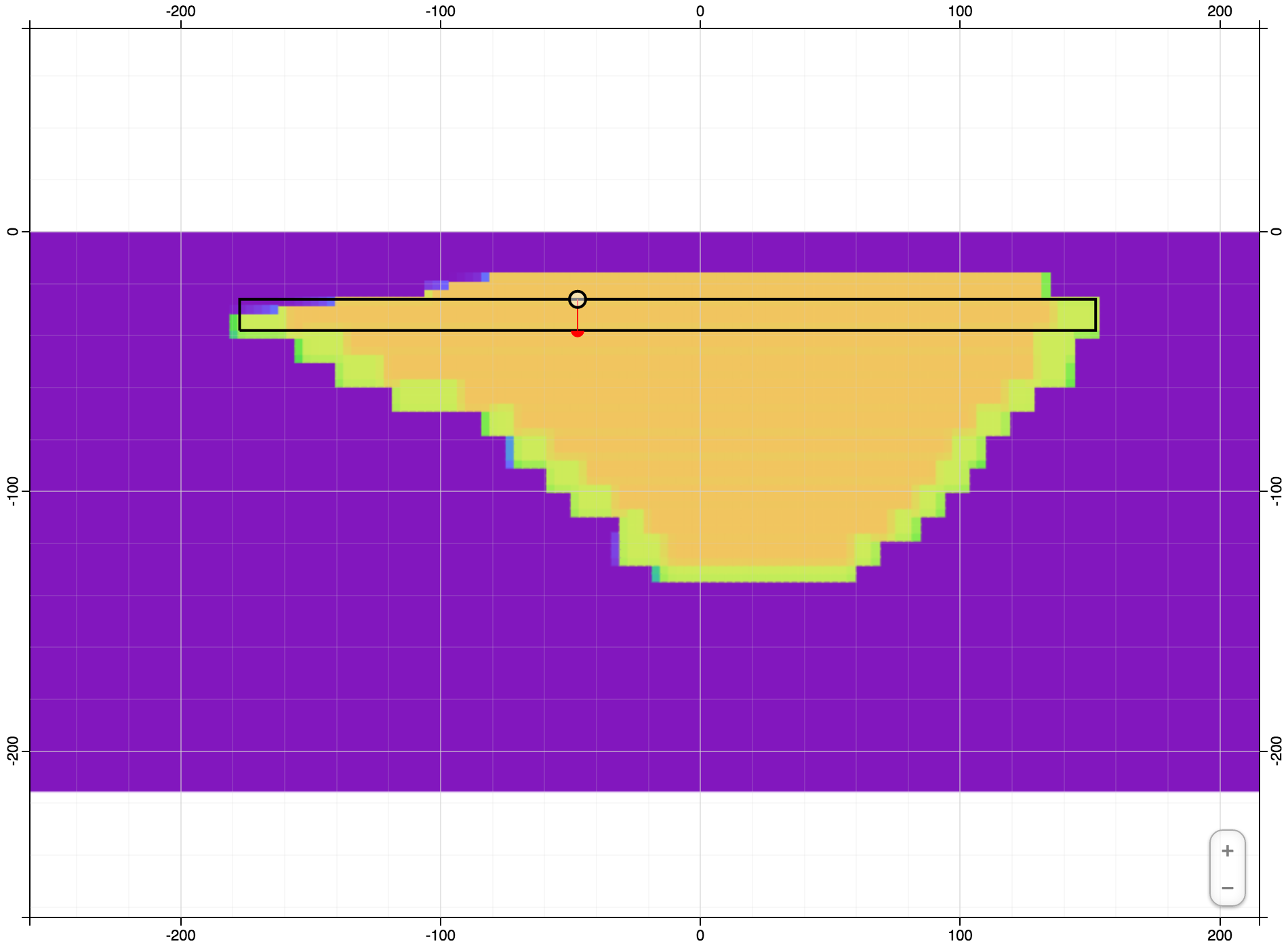
Ovoid Z-Section
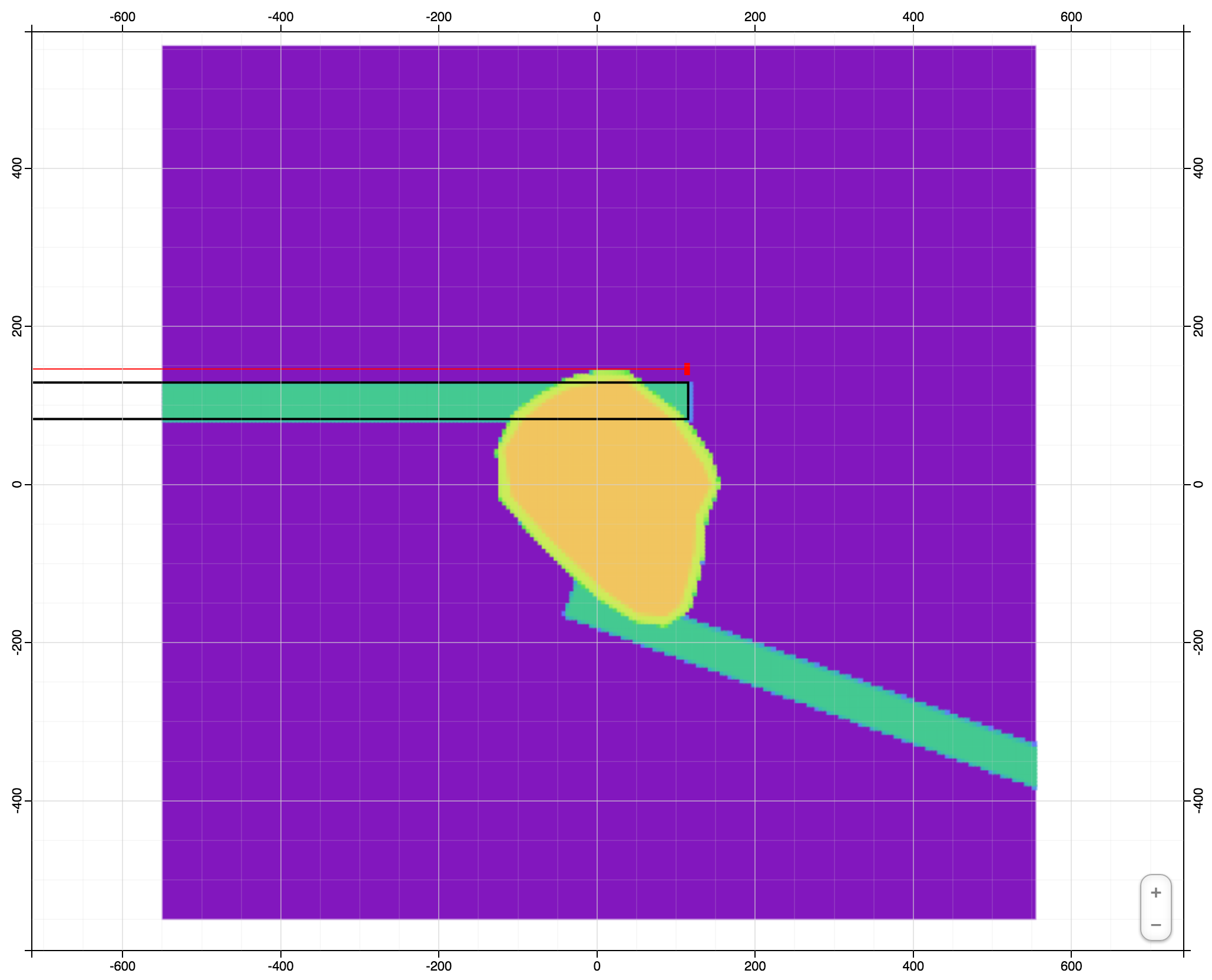
Adding OverBurden

Troctolyte Dykes - Level 1
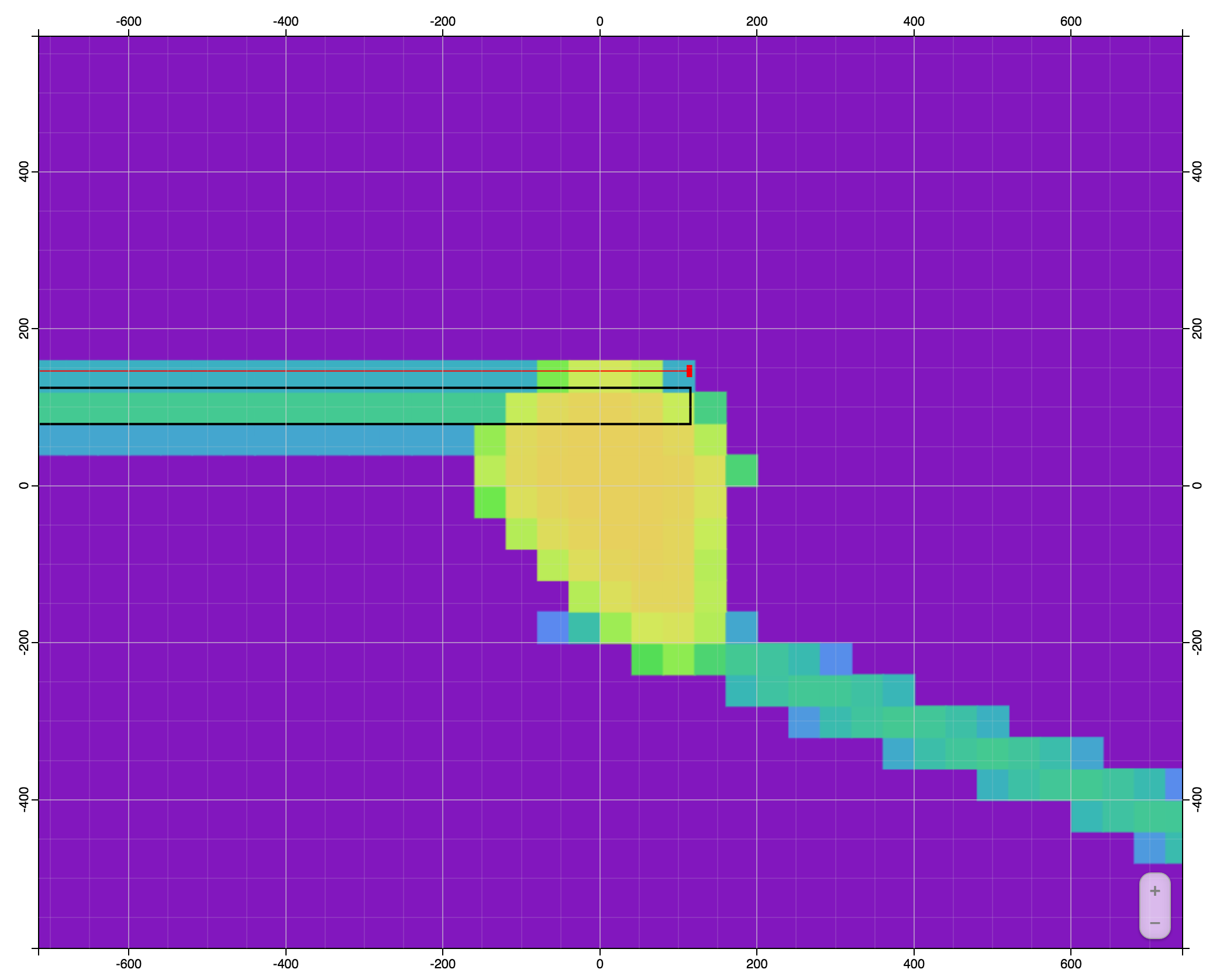
Troctolyte Dykes - Level 3
Troctolyte Dykes - Level 6
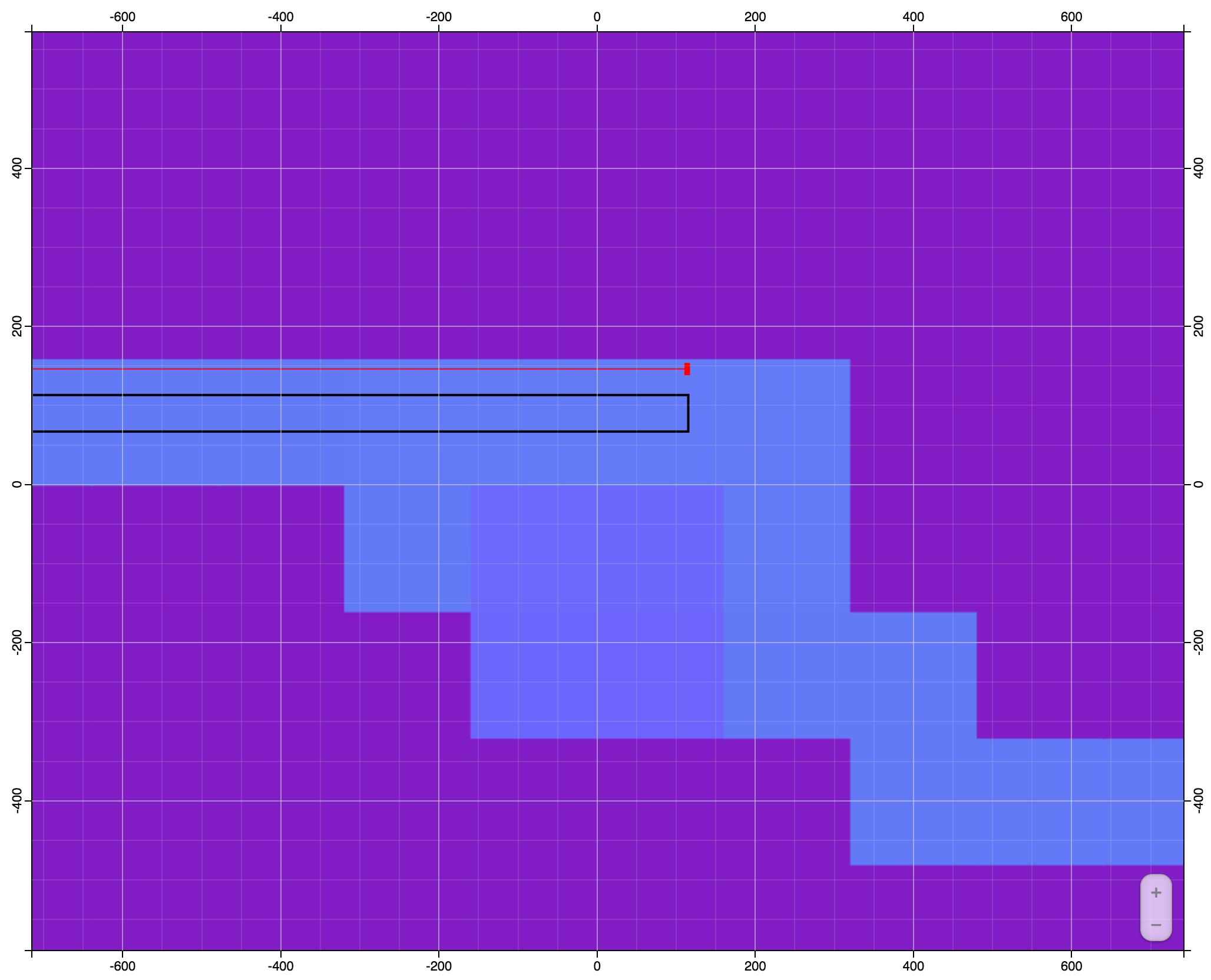
Adding a Conduit at Depth
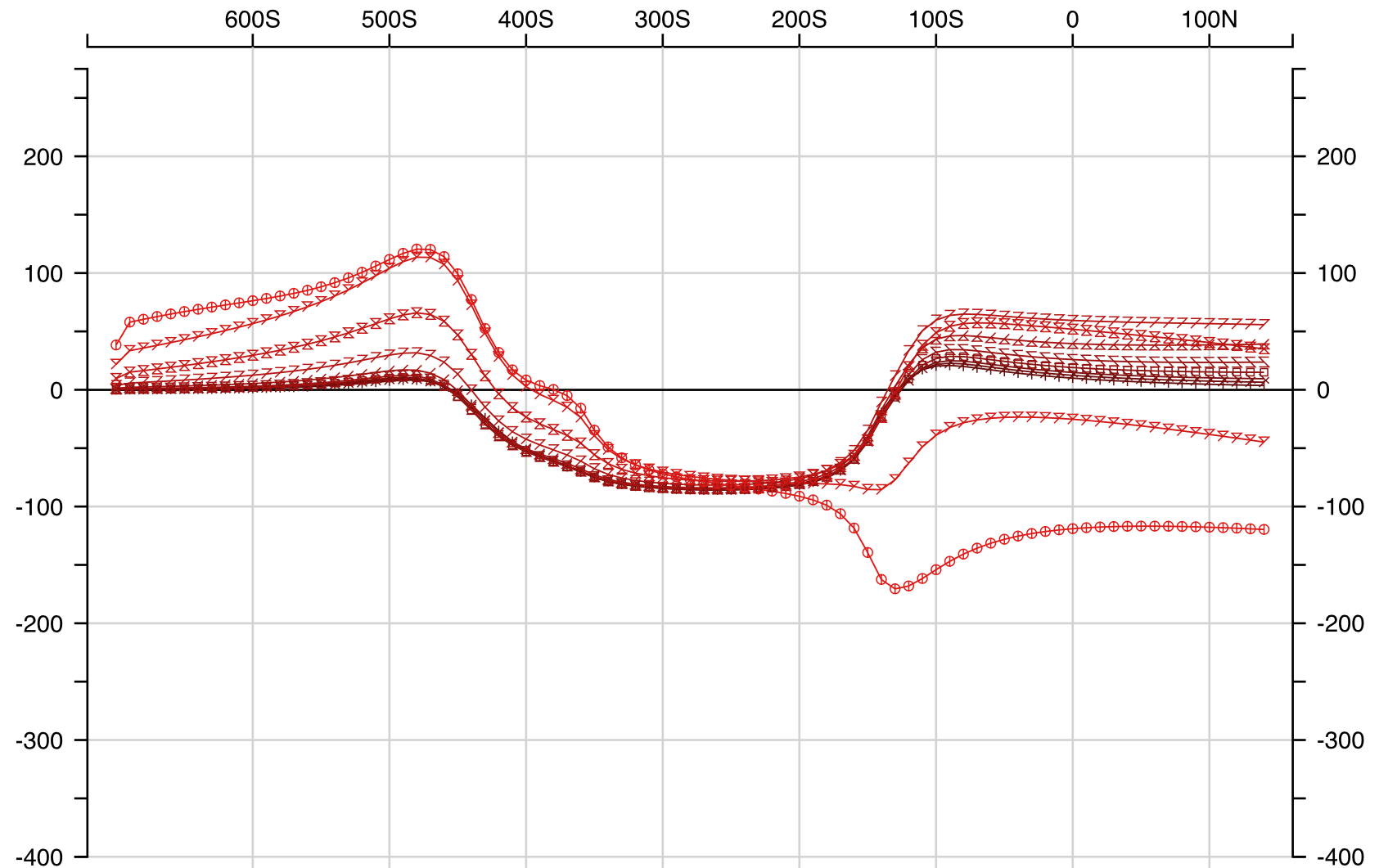
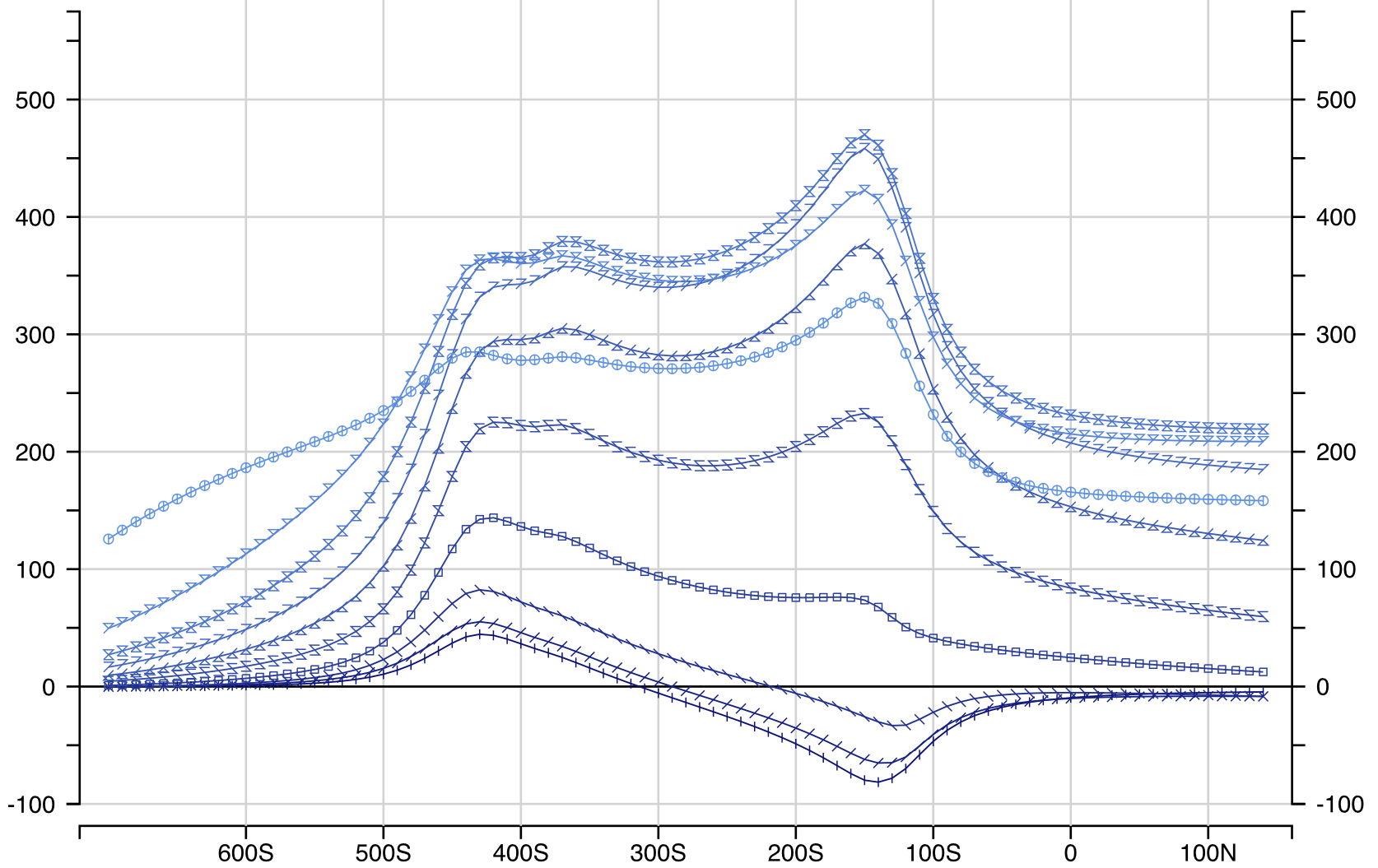
Ovoid + OverBurden Hz
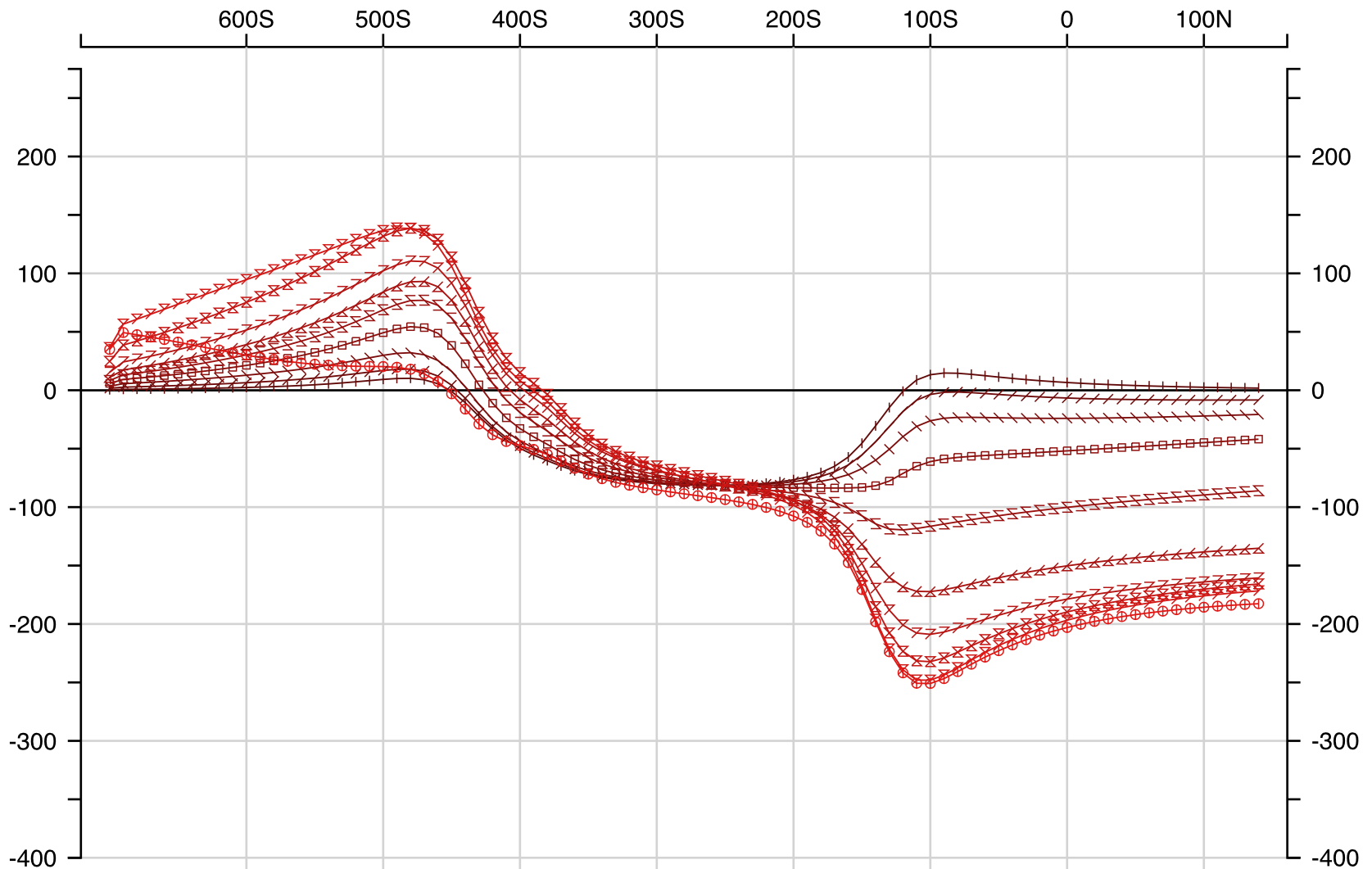
Ovoid + OverBurden + Dykes Hz
Ovoid + OverBurden + Dykes + Conduit Hz
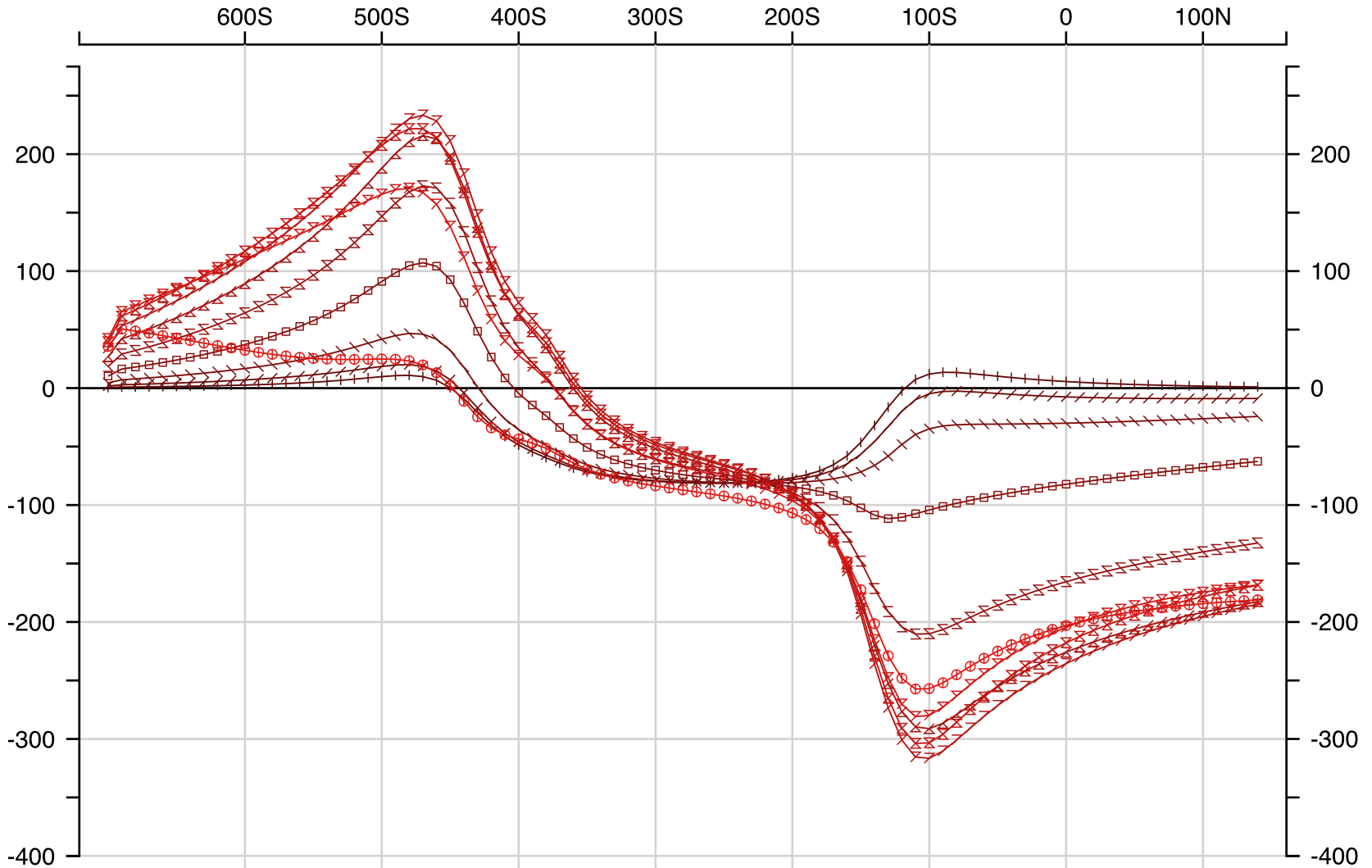
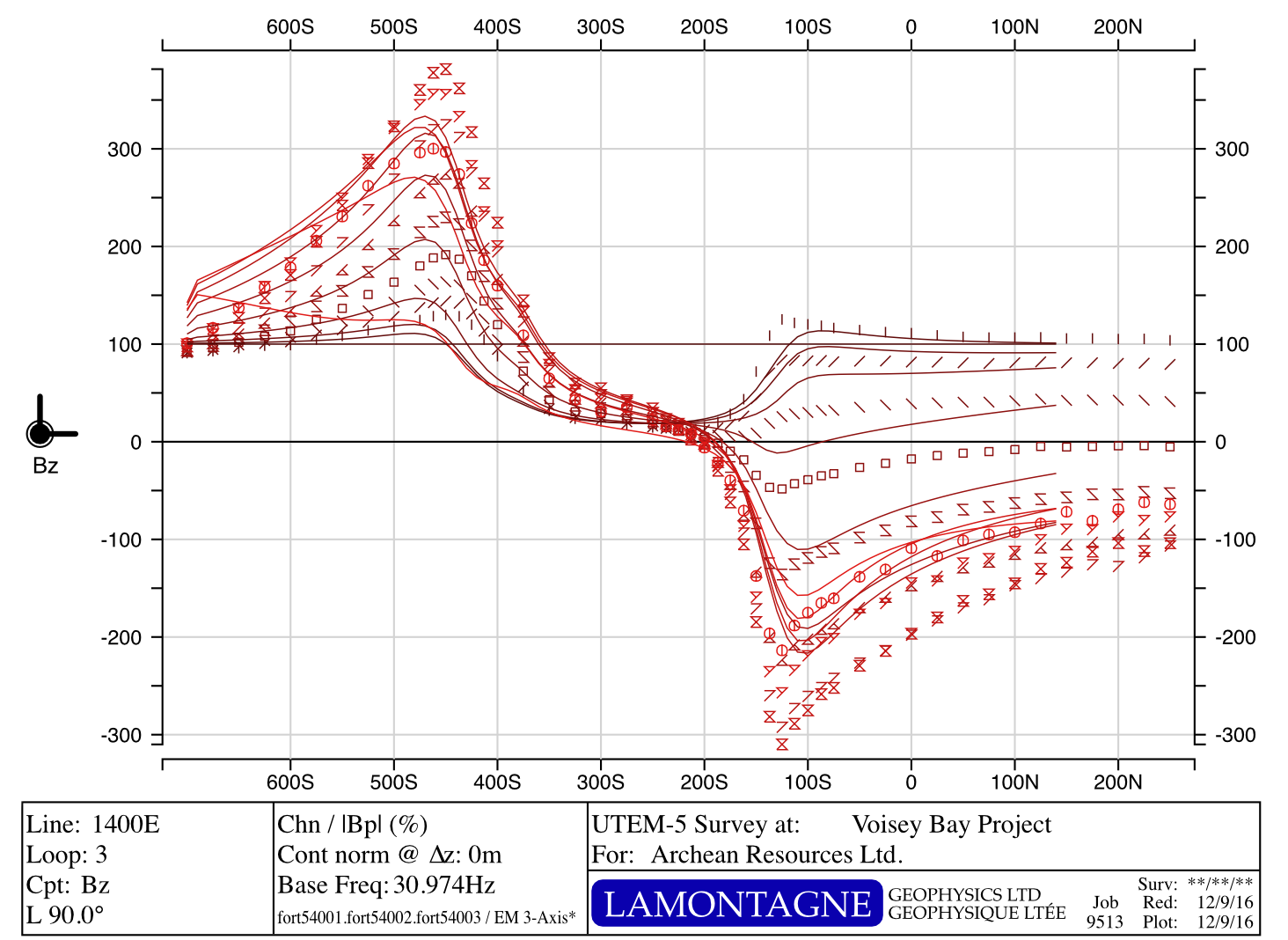
Ovoid + OverBurden Hx
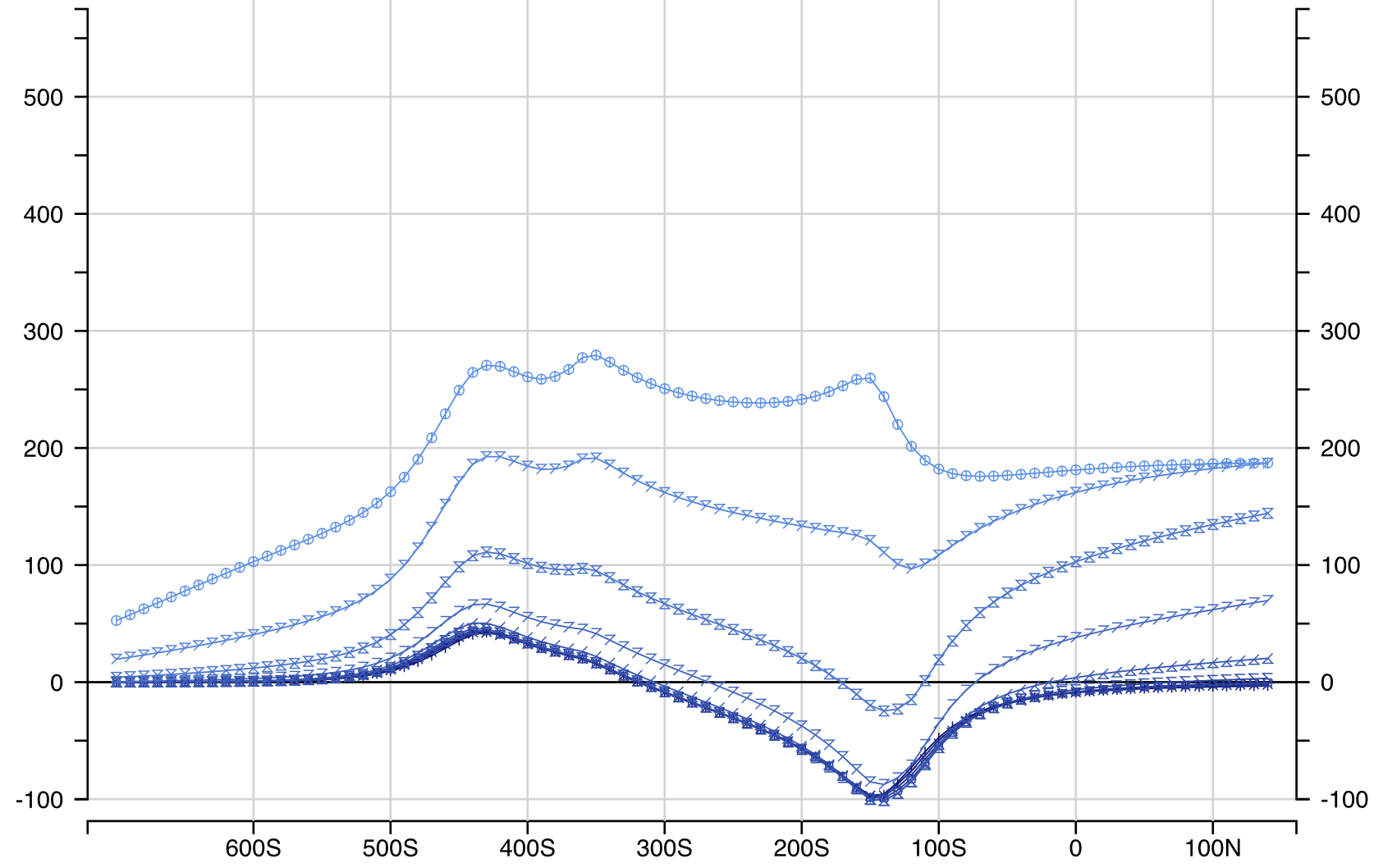
Ovoid + OverBurden + Dykes Hx
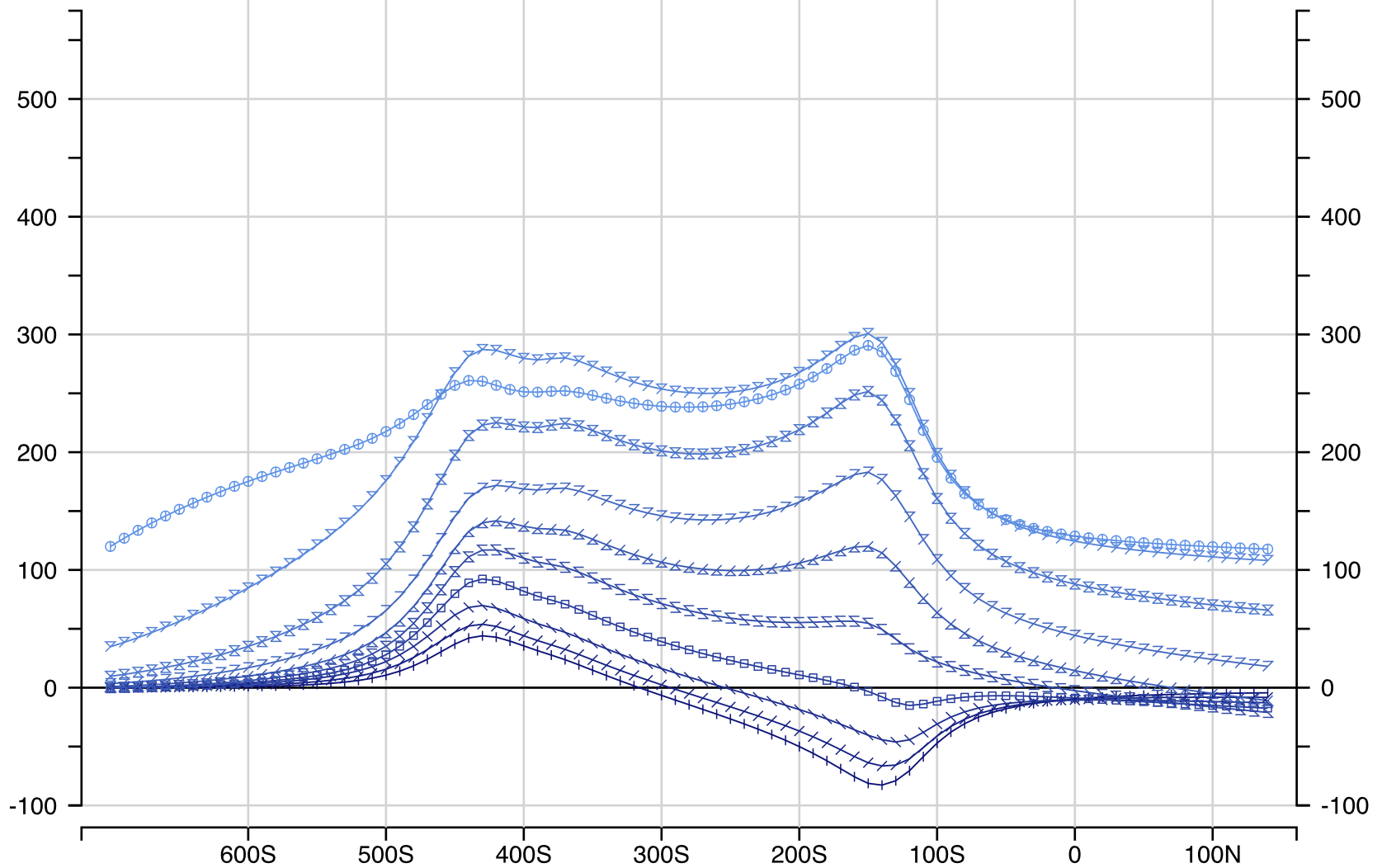
Ovoid + OverBurden + Dykes + Conduit Hx
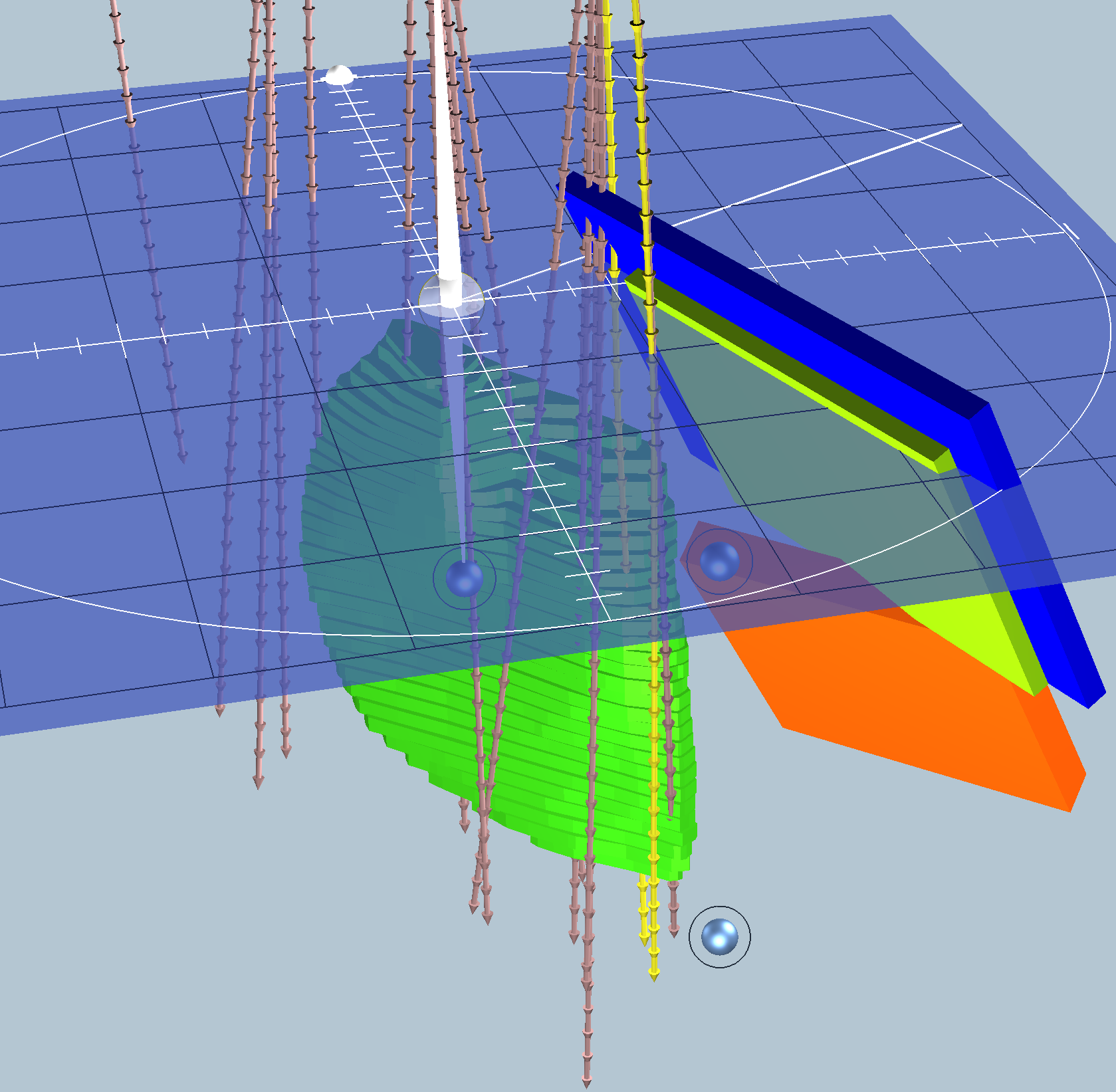
A More Realistic Exploration Example
Thank You!
References
Balch, S., 1999, Geophysical methods for nickel deposits with examples from Voisey’s Bay: GAC-MAC Meeting, St John’s Newfoundland.
Bengert, B., 2006, Successful application of electro-magnetics in the Reid Brook zone: targeting economic needles in a highly conductive haystack: ASEG Extended Abstracts 2006, 1–4.
Bochev, P. B., J. J. Hu, C. M. Siefert, and R. S. Tuminaro, 2008, An algebraic multigrid approach based on a compatible gauge reformulation of Maxwell’s equations: SIAM Journal on Scientific Computing, 31, 557–583.
Haber, E., D. Oldenburg, and R. Shektman, 2007, Inversion of time domain 3D electromagnetic data: Geophysical Journal International, 132, 1324–1335.
Hiptmair., R., 1997, Multigrid method for H(div) in three dimensions: Electronic Transactions on Numerical Analysis, 6, 133–152.
Hiptmair, R., 1999, Multigrid method for Maxwell’s equations: SIAM Journal on Numerical Analysis, 36, 204–225.
Hiptmair, R., and J. Xu, 2007, Nodal auxiliary space preconditioning in H(curl) and H(div) spaces: SIAM Journal on Numerical Analysis, 45, 2483–2509.
Jahandari, H., and C. G. Farquharson, 2014, A finite-volume solution to the geophysical electromagnetic forward problem using unstructured grids: Geophysics, 79, no. 6, E287-E302.
King, A., 2007, Review of geophysical technology for Ni-Cu-PGE deposits: Proceedings of the 5th Decennial International Conference on Mineral Exploration, 647–665.
Lamontagne, Y., and R. Langridge, 2013, UTEM ISR - induced source resistivity - Sierra Gorda project: ISR across a development-stage copper-molybdenum: KEGS PDAC Symposium 2013, 1-4.
Li, C., and A. J. Naldrett, 1999, Geology and petrology of the Voisey’s Bay intrusion: reaction of olivine with sulfide and silicate liquids: Lithos, 47, 1–31.
Lu, J. J., X. P. Wu, and K. Spitzer, 2010, Algebraic multigrid methods for 3D DC resistivity modeling: Chinese Journal of Geophysics, 53, 700–707.
Moucha, R., and R. C. Bailey, 2004, An accurate and robust multigrid algorithm for 2D forward resistivity modelling: Geophysical Prospecting, 52, 197-212.
Newman, G. A., 2014, A review of high-performance computational strategies for modeling and imaging of electromagnetic induction data: Surveys in Geophysics, 35, 85-100.
Polzer, B., 2000, The role of borehole EM in the discovery and definition of the Kelly Lake Ni- Cu deposit, Sudbury, Canada: 70th Annual International Meeting, SEG, Expanded Abstracts, 1063-1066.
Press, W. H., B. P. Flannery, S. A. Teukolksky, and W. T. Vetterling, 1988, Numerical recipes in C, the art of scientific computing: Cambridge University Press.
Yang, D., and D. W. Oldenburg, 2010, 3D forward modelling and inversion of inductive source resistivity data: 80th Annual International Meeting, SEG, Expanded Abstracts, 588-592.